-
Posts
938 -
Joined
Profile Information
-
Location
– European Union
Recent Profile Visitors
The recent visitors block is disabled and is not being shown to other users.
-
 Waldemar reacted to a post in a topic:
Age of Sail 2 - 3d ship models for PC wargame
Waldemar reacted to a post in a topic:
Age of Sail 2 - 3d ship models for PC wargame
-
 Waldemar reacted to a post in a topic:
Age of Sail 2 - 3d ship models for PC wargame
Waldemar reacted to a post in a topic:
Age of Sail 2 - 3d ship models for PC wargame
-
 Waldemar reacted to a post in a topic:
Age of Sail 2 - 3d ship models for PC wargame
Waldemar reacted to a post in a topic:
Age of Sail 2 - 3d ship models for PC wargame
-
 Glenn Cook reacted to a post in a topic:
Age of Sail 2 - 3d ship models for PC wargame
Glenn Cook reacted to a post in a topic:
Age of Sail 2 - 3d ship models for PC wargame
-
 Waldemar reacted to a post in a topic:
Age of Sail 2 - 3d ship models for PC wargame
Waldemar reacted to a post in a topic:
Age of Sail 2 - 3d ship models for PC wargame
-
 TheDukeOfWybourne reacted to a post in a topic:
HMS Falken - little sister of HMS Wasa
TheDukeOfWybourne reacted to a post in a topic:
HMS Falken - little sister of HMS Wasa
-
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
HMS Falken - little sister of HMS Wasa
Waldemar replied to Nirvana's topic in Nautical/Naval History
Yes, it looks promising in a full-fledged publication. In the past, I might have missed the design concepts for this ship, but recently, out of necessity, I have become self-sufficient in this particular matter . -
 Archi reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
Archi reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
-
 Archi reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
Archi reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
-
 Mike Y reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
Mike Y reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
-
 Hubac's Historian reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
Hubac's Historian reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
-
 Mike Y reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
Mike Y reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
-
 Waldemar reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
Waldemar reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
-
 EJ_L reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
EJ_L reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
-
 Waldemar reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
Waldemar reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
-
 GrandpaPhil reacted to a post in a topic:
Le Coureur 1776 - Shipyard 1:72-scale Laser-Cut Card Model Kit
GrandpaPhil reacted to a post in a topic:
Le Coureur 1776 - Shipyard 1:72-scale Laser-Cut Card Model Kit
-
 catopower reacted to a post in a topic:
Le Coureur 1776 - Shipyard 1:72-scale Laser-Cut Card Model Kit
catopower reacted to a post in a topic:
Le Coureur 1776 - Shipyard 1:72-scale Laser-Cut Card Model Kit
-
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Review Le Coureur 1776 - Shipyard 1:72-scale Laser-Cut Card Model Kit
Waldemar replied to catopower's topic in REVIEWS: Model kits
There is another explanation, even more likely. The differences in the hull shapes on both plans from that era are due to the fact that although the British were able to measure the hulls of the captured vessels very accurately, already when drawing up their plans, they were, in a sense, designing such vessels from scratch, but using different design methods, which inevitably altered the actual shapes of the hulls to a greater or lesser extent. Not to mention the natural deformations of the hull during the ship's use. This is why today's French researchers consistently reject the lines from such plans of captured ships, preferring instead the original plans by the French designers (if, of course, they have a choice). And rightly so, and this is the first exception to this rule that I am aware of. For the above reasons, the original French design plan should be considered more reliable in terms of the shape of the hull, rather than an approximation made after the ship's capture using other design techniques that are incompatible with the original ones.- 12 replies
-
- Le Coureur
- Shipyard
-
(and 1 more)
Tagged with:
-
Either way, from what you have just shown, there is still a lot of dubbing to be done on normal frames. Just like in real-scale construction!
- 119 replies
-
- ancre
- Bateau de Lanveoc
-
(and 2 more)
Tagged with:
-
Hi Jacques, I've been following your thread and I can see that lately it's been an unnecessary road through frustration leading to disappointment . If I may suggest, shape the surfaces of both ends of the hull in the same way as the original builders did. Start by removing the cant frames (although one pair at the stern may still prove useful, choose one of them in this case). Prepare and attach 5 or 6 ribbands/battens per side. Keep an eye on symmetry, especially at the ends of the hull, but a slight asymmetry will not be the end of the world, just as it was not in real vessels. Only then shape and secure the cant frames, guided by the battens, preferably using temporary paper templates. Alternatively, instead of battens, you can try normally planking the hull right away, and only then shape and attach the cant frames, already more to reinforce the structure than to shape the surface. What do you think?
- 119 replies
-
- ancre
- Bateau de Lanveoc
-
(and 2 more)
Tagged with:
-
 Waldemar reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
Waldemar reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
-
 Waldemar reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
Waldemar reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
-
Intentional skewing of the bowsprit and beakhead seems rather unlikely, as the bowsprit runs exactly over the stempost (unlike, for example, English designs of the period, where the bowsprit ran alongside the stempost, always or at least often) and there was no reason to angle them in the horizontal plane. This arrangement is particularly evident in Björn Landström's fantastic illustrations in his equally fantastic book The Royal Warship Vasa, published in 1988. I highly recommend consulting this publication as well.
-
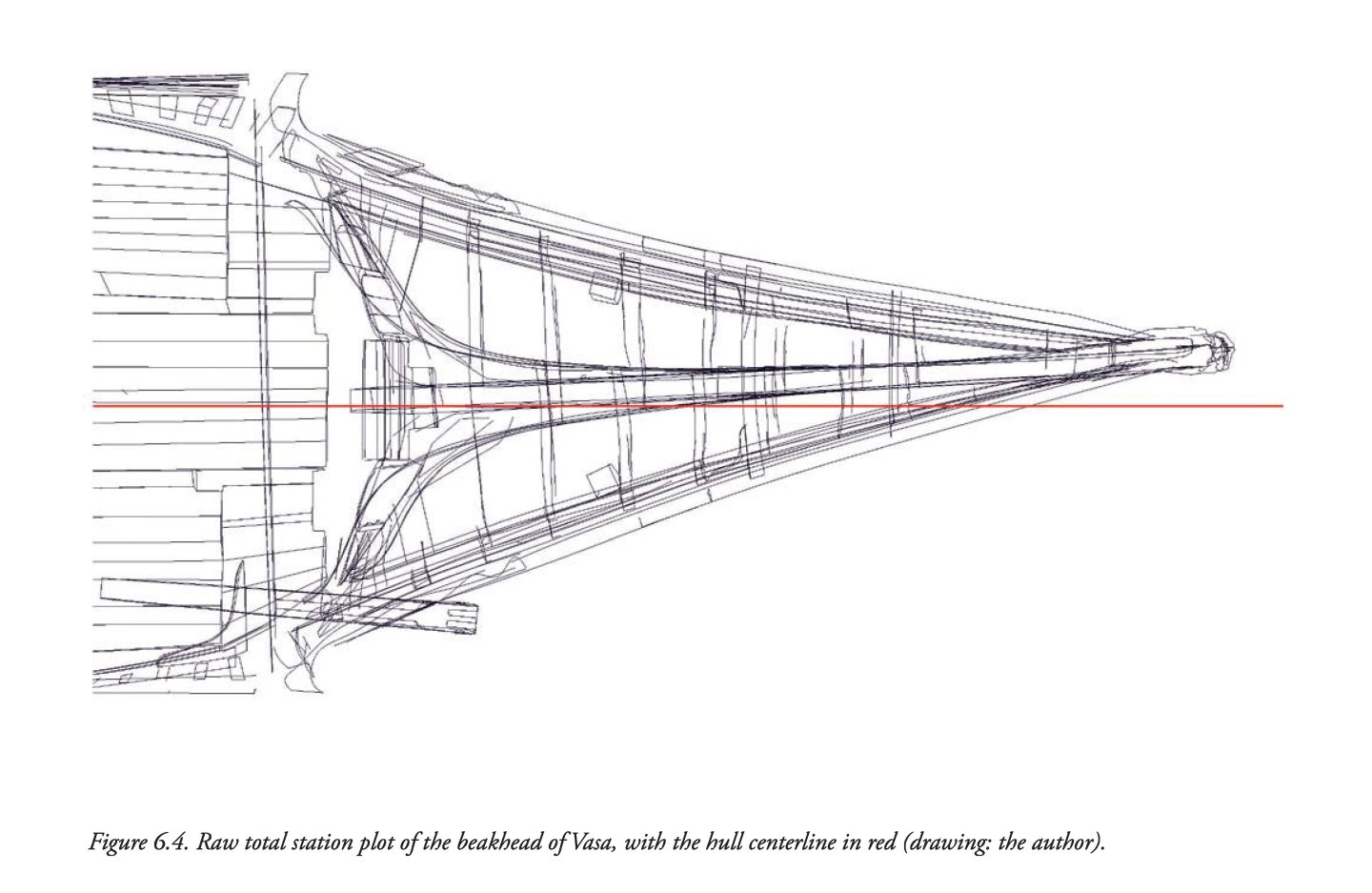
This will probably be the first model of the Vasa 1628 that I know of that will (intentionally) incorporate all the known defects and flaws of this vessel. I am particularly curious to see how the distortion of the beakhead will look in the photos of the model. It can be said that this rather conspicuous feature is never reproduced by model makers, so I commend the courage to do so. Not everyone may know what this is about, so below is an illustration showing the beakhead distortion as it appears on the original (from a paper by Fred Hocker, In Details Remembered. Interpreting the Human Component in Shipbuilding, 2013). In fact, this very feature was omitted even in the official plans of the ship published in 1980, and there is still no updated set of plans.
-
As for your fundamental decision to build a model with all the flaws of the original, I actually agree with it and it would probably be my choice as well. I think I would even make a crooked beakhead like in the original, instead of a straight one. On the other hand, my diagnosis of the causes of these errors would be different. For example, it is known that the chief designer's successor realised during construction that the design was flawed and tried to remedy it, but unsuccessfully, and even these ‘rescue’ attempts introduced further errors with disastrous consequences. As it happens, other designers and builders at that time did not normally make these mistakes.
-
 Waldemar reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
Waldemar reacted to a post in a topic:
Vasa 1628 by EJ_L - Scale 1:24 - Fully Scratch Built and Large!
-
Hello E.J., In general, the flaws in the shape of the Vasa 1628 hull can be divided into two categories. The first category results from incorrect proportions, in particular the dramatically insufficient depth in hold. And in this category, there is rather nothing that can be done (corrected) when building the model. However, the second category is more subtle, because the ship was ultimately built quite sloppily in terms of geometric correctness as well. This is especially expressed in the inappropriate and at the same time irregular course of the actual maximum breadth line. As shown in the diagram below in blue dashed line. I simply have a question: are you going to ‘normalise’ the defects from this second category, or will you try to reproduce them in your model after all?
-
Thukydides started following Waldemar
-
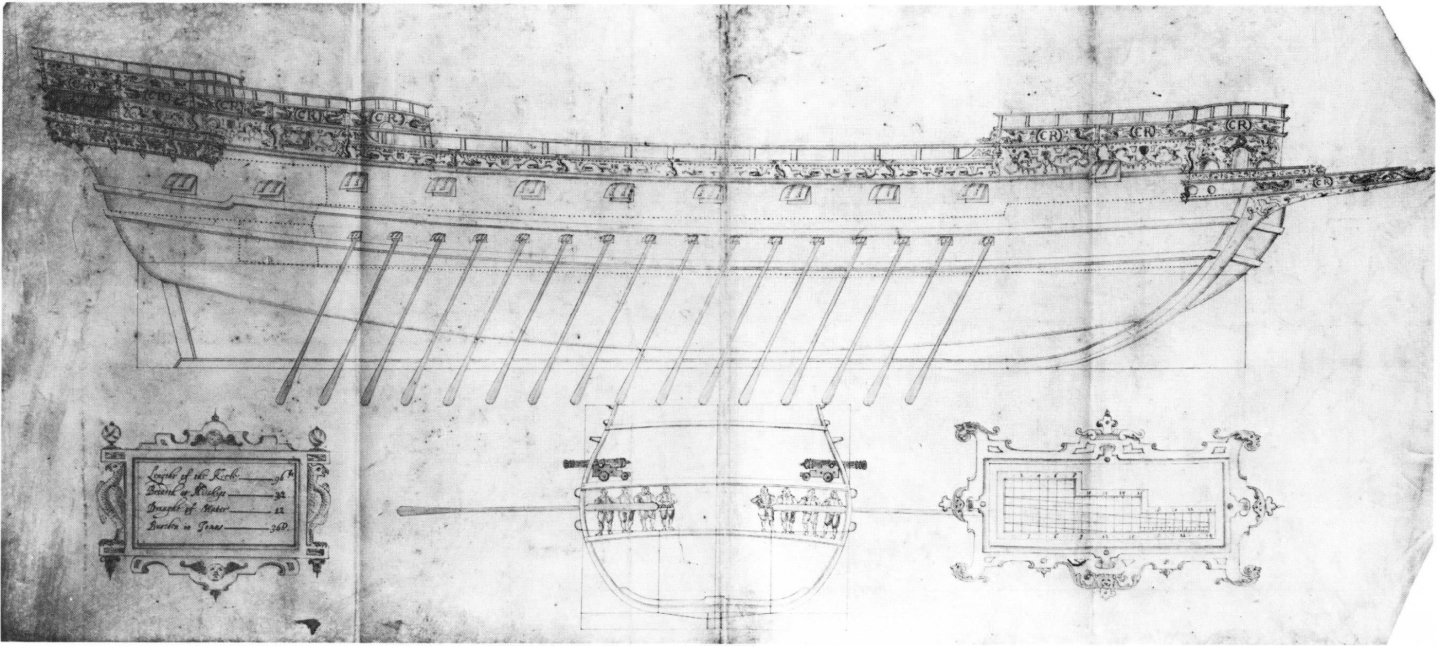
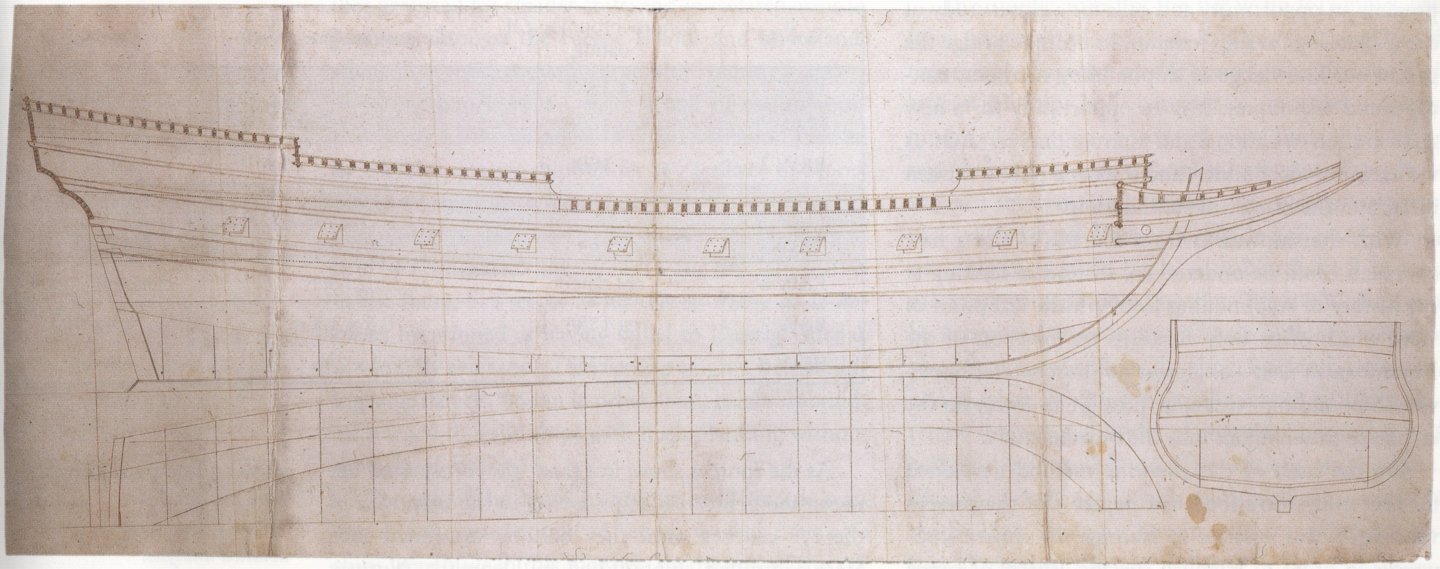
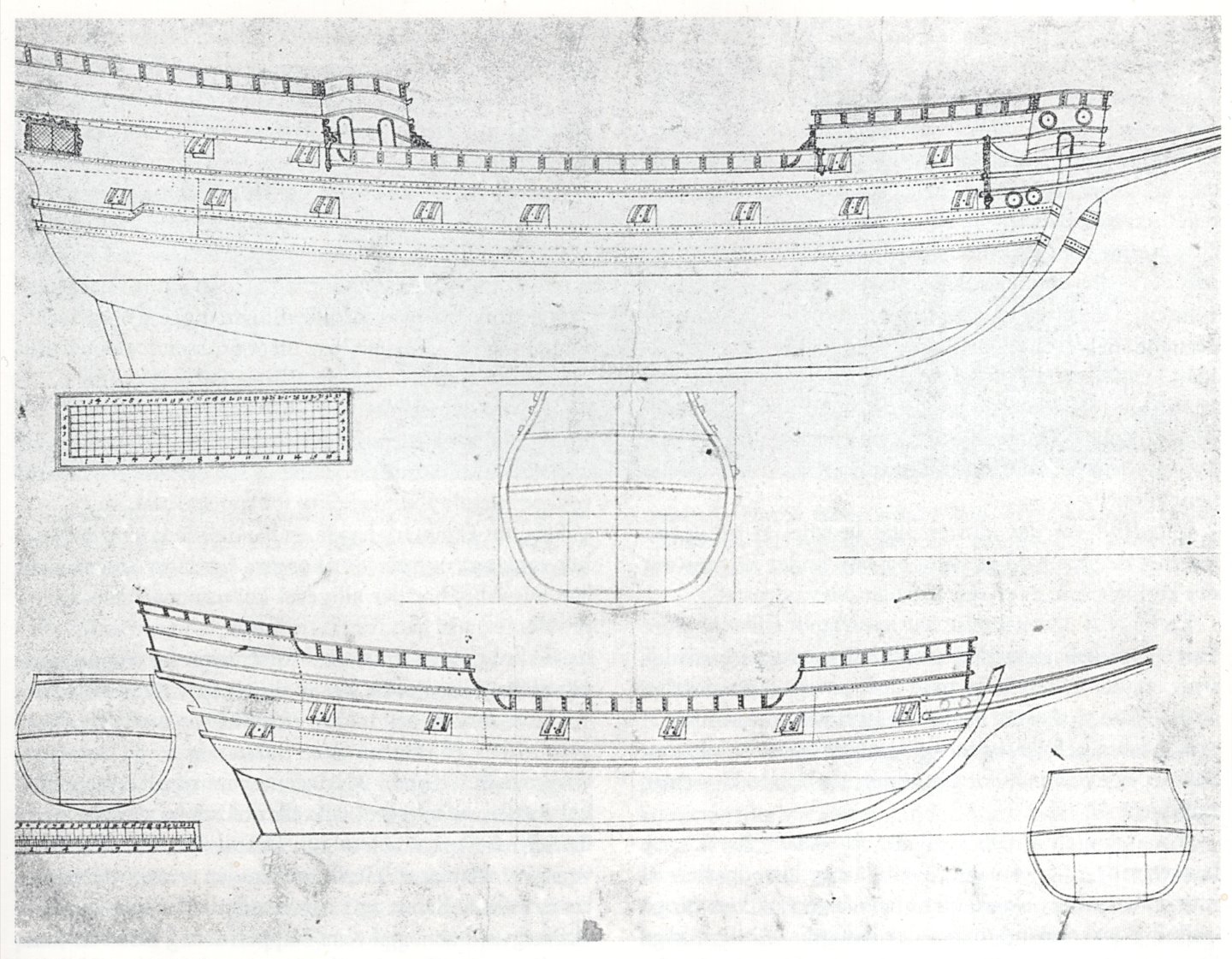
Once again, I am reviewing and analysing Dudley's vast, unpublished material and wondering how best to use, comment on and describe it. There is so much diverse information, some more important than others, that the decision on the best possible course of action is not at all obvious. In the meantime, it is worth presenting some extant designs by British (English and Scottish) designers from that period, taken from Danish and British archives, which share the same Mediterranean design paradigm as Dudley's designs — in addition to longitudinal design lines (risings and narrowings; yet no line of the greatest breadth, just ‘boca’ line instead), there is also a master frame mould. No other frames and no auxiliary design lines such as waterlines, buttock lines or diagonals. Project ca. 1600 Project ca. 1600 Project ca. 1600 Project ca. 1625 Project 1623
-
Thank you. Quite recently, I even started a thread on this very issue on a French-language forum, but before I got to the details and the heart of the matter, circumstances led me to decide to continue on one of the English-language forums. The continuation was supposed to be immediate, but somehow Mary Rose 1511 and Dudley got in the way . But that's even better, because it all ties together, so chronologically it's more advantageous. Either way, it needs to be revisited, and now I plan to do so after Dudley.
- 2,671 replies
-
- heller
- soleil royal
-
(and 9 more)
Tagged with:
-
Ah, line drawings are just a derivative; it's all about naval architecture and its evolution, the image of which is completely distorted today.
- 2,671 replies
-
- heller
- soleil royal
-
(and 9 more)
Tagged with:
-
Admittedly, structural aspects are rather secondary to me, so they are not my main focus, but criticism of various solutions adopted in these reconstructions can be found on the website http://www.gerard-delacroix.fr/. However, I have serious reservations about the design concepts used in the 17th-century reconstructions. But this is a broader topic, requiring a whole thread with explanations and examples...
- 2,671 replies
-
- heller
- soleil royal
-
(and 9 more)
Tagged with:
-
Okay, thank you very much, I just wanted you to see it as it appears in the source iconography. And of course, you decide for yourself what to do with it. Incidentally, to be honest, I have serious issues with some aspects of Lemineur's reconstructions, and I'm not the only one. Boudriot's work is much more convincing, so to speak.
- 2,671 replies
-
- heller
- soleil royal
-
(and 9 more)
Tagged with:
About us
Modelshipworld - Advancing Ship Modeling through Research
SSL Secured
Your security is important for us so this Website is SSL-Secured
NRG Mailing Address
Nautical Research Guild
237 South Lincoln Street
Westmont IL, 60559-1917
Model Ship World ® and the MSW logo are Registered Trademarks, and belong to the Nautical Research Guild (United States Patent and Trademark Office: No. 6,929,264 & No. 6,929,274, registered Dec. 20, 2022)
Helpful Links
About the NRG
If you enjoy building ship models that are historically accurate as well as beautiful, then The Nautical Research Guild (NRG) is just right for you.
The Guild is a non-profit educational organization whose mission is to “Advance Ship Modeling Through Research”. We provide support to our members in their efforts to raise the quality of their model ships.
The Nautical Research Guild has published our world-renowned quarterly magazine, The Nautical Research Journal, since 1955. The pages of the Journal are full of articles by accomplished ship modelers who show you how they create those exquisite details on their models, and by maritime historians who show you the correct details to build. The Journal is available in both print and digital editions. Go to the NRG web site (www.thenrg.org) to download a complimentary digital copy of the Journal. The NRG also publishes plan sets, books and compilations of back issues of the Journal and the former Ships in Scale and Model Ship Builder magazines.