-
Posts
938 -
Joined
Content Type
Profiles
Forums
Gallery
Events
Everything posted by Waldemar
-
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Jesus Boat ... Rhino
Waldemar replied to tabycz's topic in CAD and 3D Modelling/Drafting Plans with Software
Impressive! Clearly now you have made the arrangement of the frames more realistic by dividing them into floors and futtocks. Just wondering, how it compares with the archaeological documentation, as I do not know the Wachsmann's monograph, just pictures of other models found on the internet. My guess is that the frame layout is somewhat idealised (i.e. made more regular), as I did in my reconstruction. -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Grinding your own lathe tools?
Waldemar replied to CPDDET's topic in Modeling tools and Workshop Equipment
Chances are you already have miniature wood cutters like the ones pictured. There is also a chance that one of them is already suitable for your needs. These cutters have usually round shanks, but then either a V-type tool holder or a regular one with an additional V-grooved shim (both pictured) will suffice. Just an idea for a painless improvising. -
Not quite sure what kind of references you are looking for, in English or in French, modern works or source material, modelling manuals or otherwise. In any case, below is a list of source works, taken (from the rather compact) modern modelling handbook Le gréement des navires anciens (1700–1850). Traité de modélisme naval by Gérard Piouffre, 2005. However, working with Gérard Delacroix's excellent monographs, you may not need to consult the contemporary written works at all, but only the detailed photographs of period models that you will find primarily in these two albums by Jean Boudriot and Hubert Berti: – Modèles historiques au Musée National de la Marine, tome I, 1997 (in French, English and Italian), – Modèles historiques au Musée National de la Marine, tome II, 2006 (in French and Italian). For more on these albums, see the Ancre website.
-
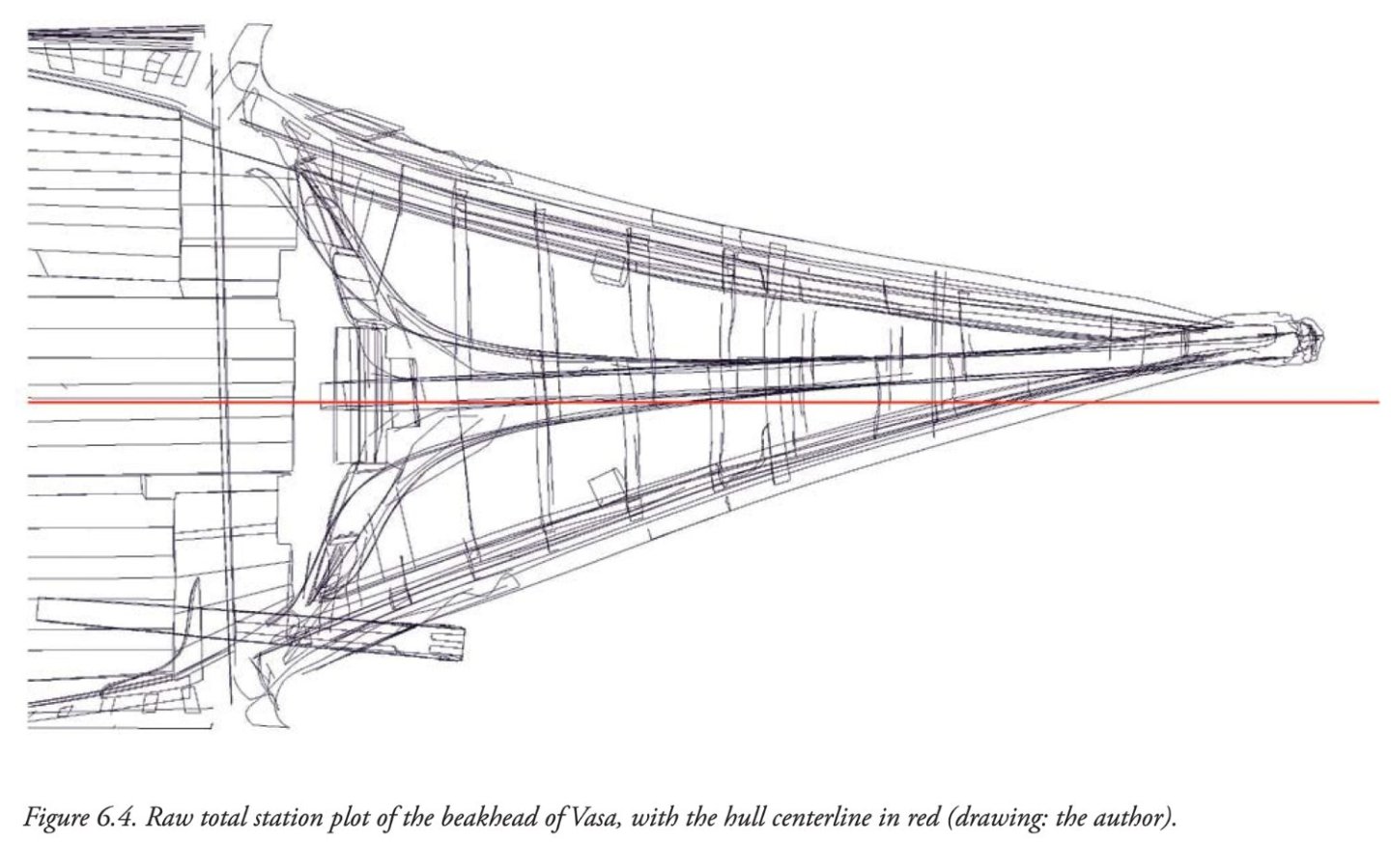
This misalignment is perhaps the most spectacular (by the same author as above). Who dares to make an accurate model of the original? 🙂
-
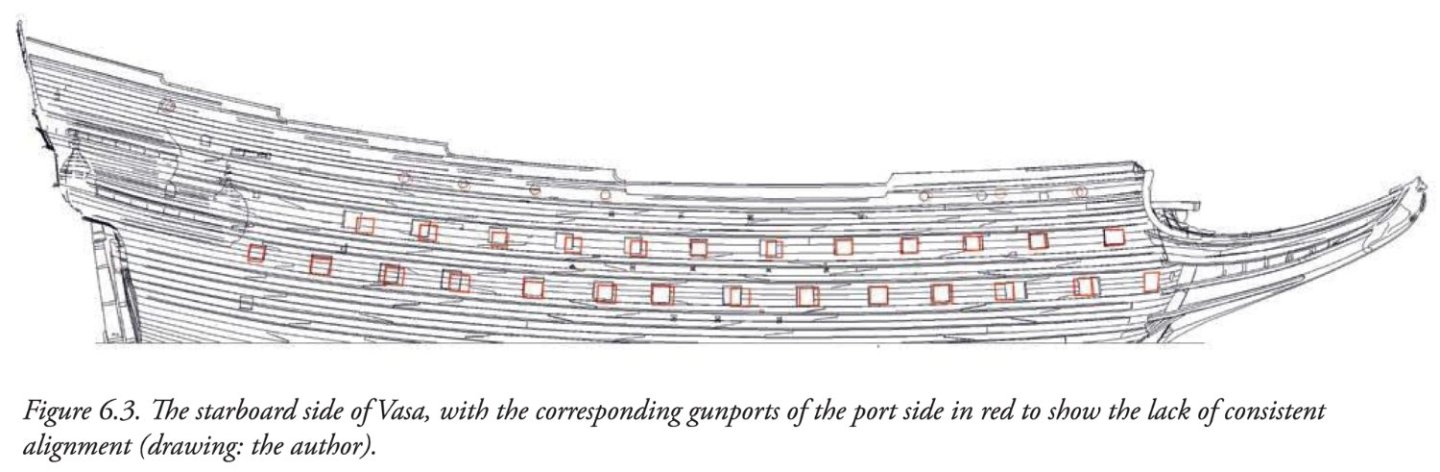
Not quite sure what you mean by 'not lining up', but please take a look at comparison of the two sides of the real Vasa by Fred Hocker (from his paper In Details Remembered. Interpreting the Human Component in Shipbuilding, 2013). There are more such 'misalignments' in the real ship, which would perhaps disqualify the model in modern modelling competitions. 🙂
-
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mary Rose – an English ship of the Mediterranean concept
Waldemar replied to Waldemar's topic in Nautical/Naval History
Yes, it is one of the best monographs in existence. For me, of course, except for the interpretation of the hull design method. -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mary Rose – an English ship of the Mediterranean concept
Waldemar replied to Waldemar's topic in Nautical/Naval History
In conclusion, for the above reasons, quite typical Mediterranean features were not recognised by the researchers of the Mary Rose wreck. Instead, some unspecified 'method', apparently not even suitable for reproducing the shape of the ship's hull, was hatched, which you seem to refer to in your post as 'other design system'. Wayne, I'm very interested in this subject, so I'd normally ask you for the details of this 'other design system' you mention (archaeological, written, design principles, whatever), but should I? -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mary Rose – an English ship of the Mediterranean concept
Waldemar replied to Waldemar's topic in Nautical/Naval History
Wayne, in truth, I prefer to talk about specific issues rather than at the level of general considerations, which are unlikely to lead to firmer conclusions. First of all, I do not, as you suggest, discount the work of previous researchers. On the contrary, I believe that familiarity with their work is even mandatory. As it happens, however, for the authors of the Mary Rose monograph, their interpretation of the hull shape of this particular ship is a key and probably the only relevant argument for some mysterious local conceptual tradition of frame-first shipbuilding method, albeit one previously unknown in the north of the continent. A sort of Deus ex machina. Unfortunately, in analyzing the shape of the hull they made, in my opinion, the fatal mistake of assuming that "the Ibero-Mediterranean method was based on tilting the frame outward from a pivot point [placed only] at the bilge", rather than placed elsewhere. This may also be related to the incomplete definition given in the study pointed out by Steven: "The smoothing-out of the kink is called cancomo in Portuguese, desfaldar in Spanish and recalement in French". However, according to one of the source definitions, recalement is done "à faire répondre la façon du bas de l'estamenaire avec le façon du madier, en diminuant sa grande rondeur et la réduisant par une juste proportion", effectively moving the pivot point beyond the contour of the frame. The description left by Lavanha also leaves no doubt that the futtock template was not simply tilted but slided along the arc of the bilge. But even taking your approach, i.e. of the "two pieces may or may not be related" kind, the degree of probability of both interpretations is quite obvious to me. So, it's not going to be easy for the authors of the Mary Rose monograph, or anyone else for that matter, to convince me of their conjecture, when they haven't even been able to present a coherent or convincing conception of the formation of the whole shape of the Mary Rose hull, as I have done. -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mary Rose – an English ship of the Mediterranean concept
Waldemar replied to Waldemar's topic in Nautical/Naval History
Hi Steven, thank you for your input. Well, I don't quite agree with the statements in this publication, and for me both the diagram and the text in this paper are more or less misleading. It says "the English method achieves the modification of the three tangent arcs by adjusting the length of adjacent cords, known as 'hauling down' and 'hauling up' the arcs". However, this is a reversal of the process. First the arcs were brought into tangency, and only then was the resulting length of the arcs read and marked on the templates. And also: "the Ibero-Mediterranean method was based on tilting the frame outward from a pivot point at the bilge" and a little further "the Ibero-Mediterranean texts used a limited version of "hauling down" the bilge arc in order to fair out the kink left at the bilge by the outward tilting of the futtock mould". He probably had in mind the description left by Lavanha, but a careful reading of this source text is not consistent with such an interpretation. Methods known from English manuscripts and publications from ca. 1600–1670 allow pre-designed frames to be formed along almost the entire length of the hull, whereas for Mary Rose to achieve this effect in some regular way is only possible for the central part of the hull. The frames (bends) outside the midship part already elude such regular methods, so they had to be shaped with flexible ribbands. This feature also fits perfectly with the Mediterranean methods. So, if we have historical information that Henry VIII imported Mediterranean shipwrights to build his fleet, why invent some implausible thesis about local methods originating from no one knows how or where? -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mary Rose – an English ship of the Mediterranean concept
Waldemar replied to Waldemar's topic in Nautical/Naval History
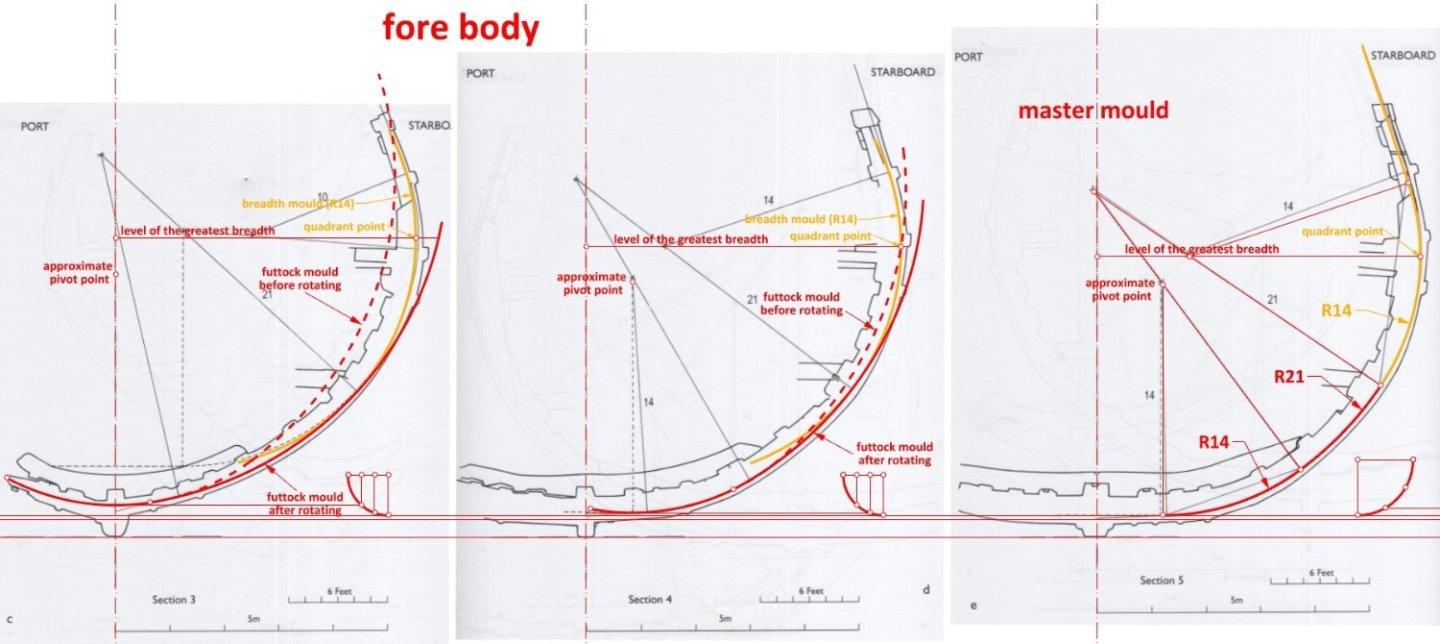
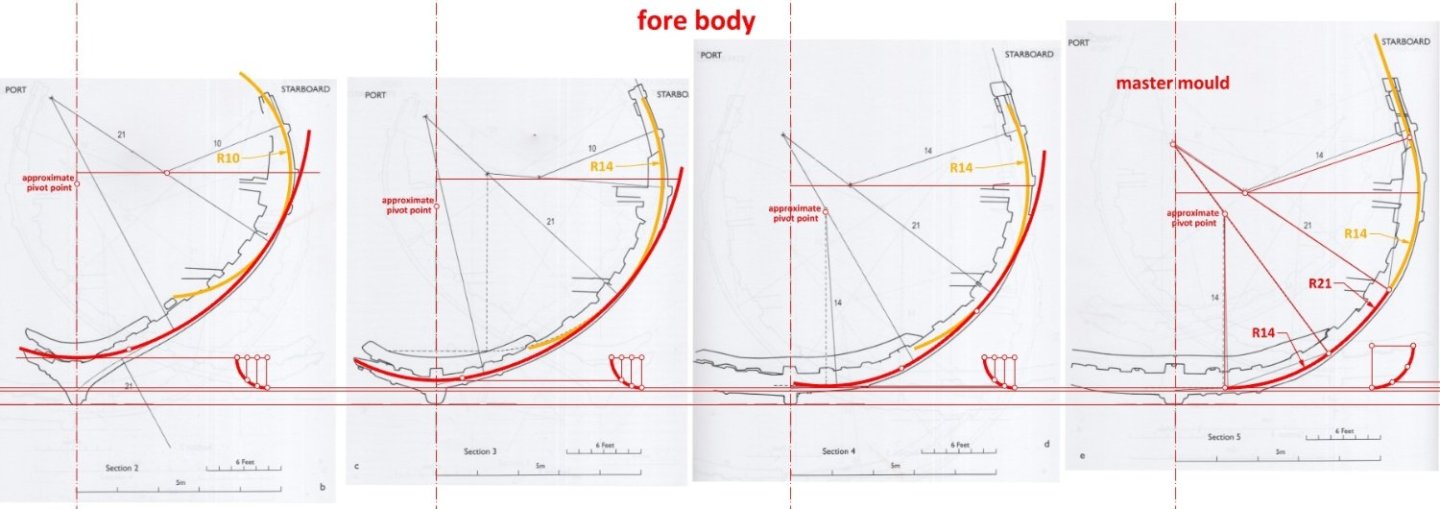
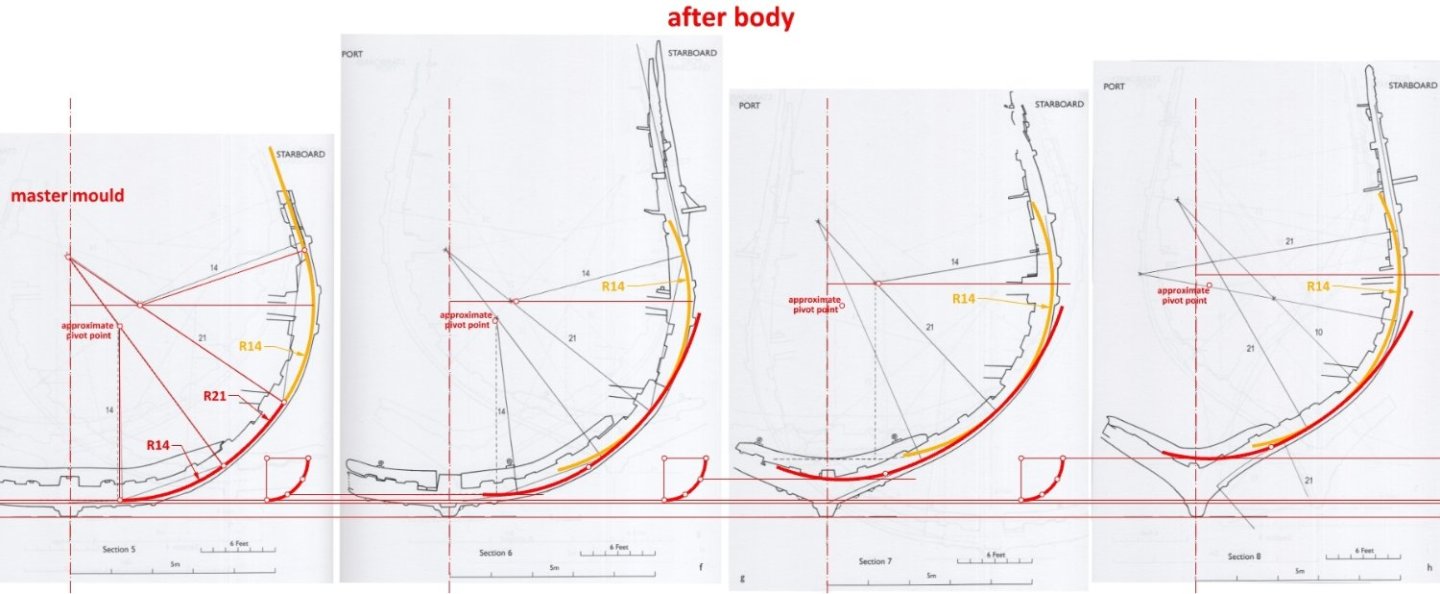
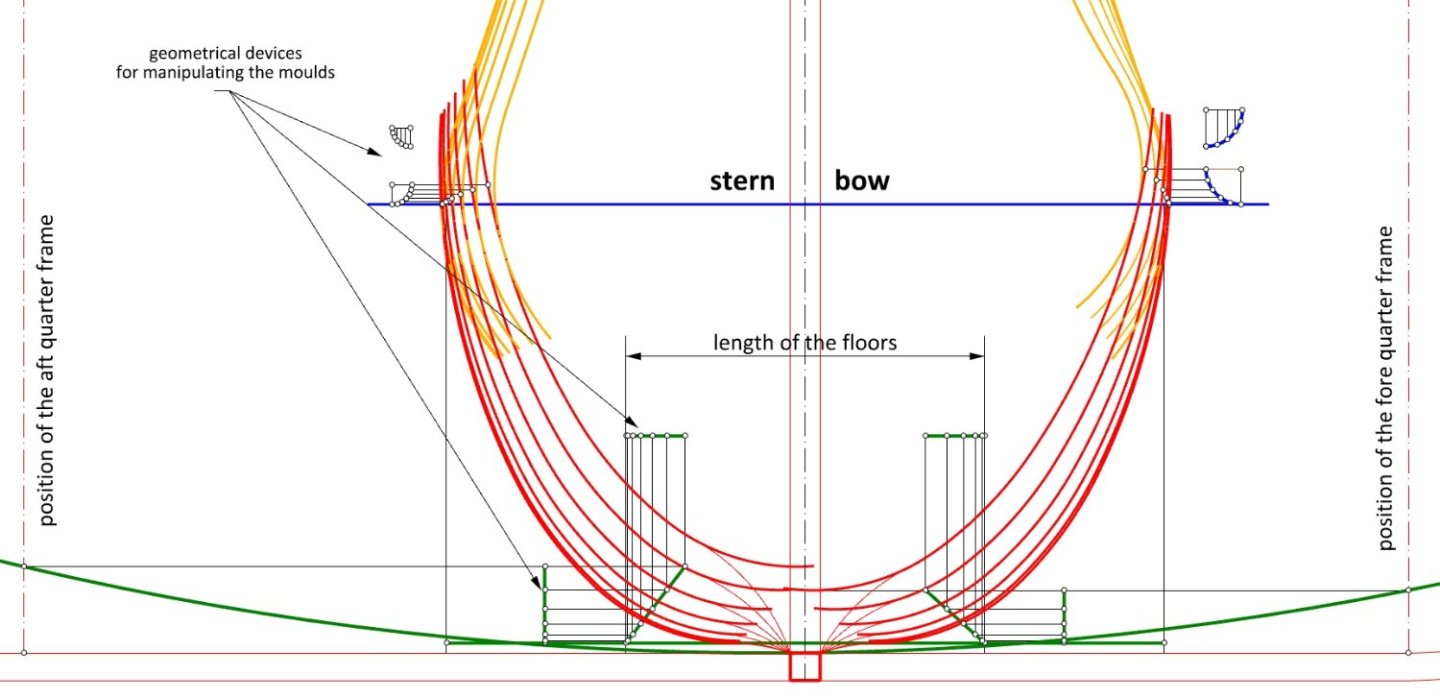
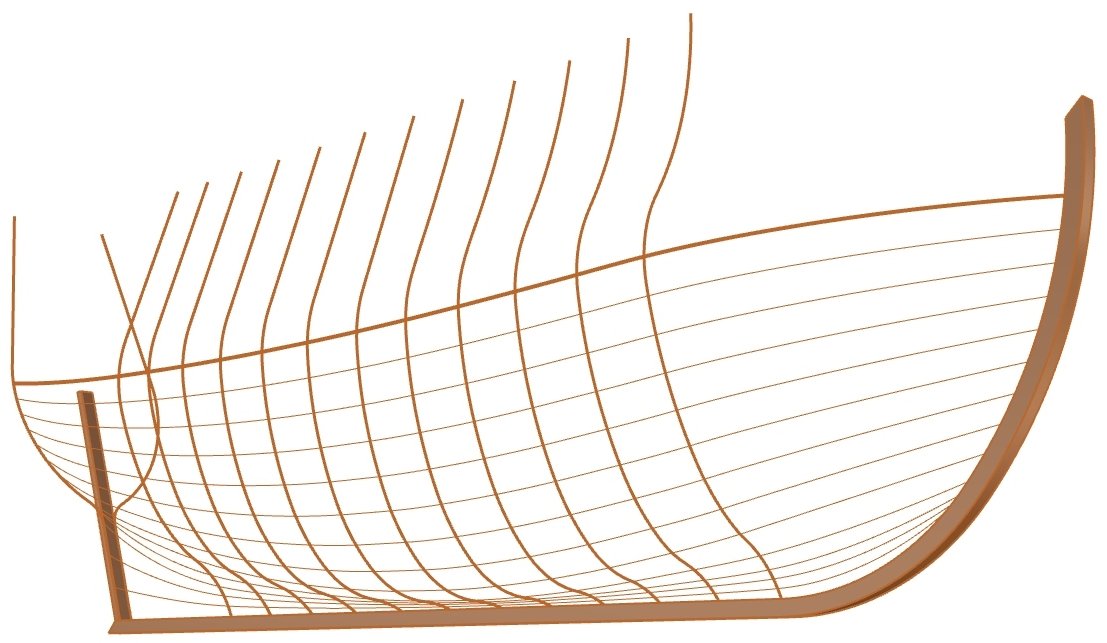
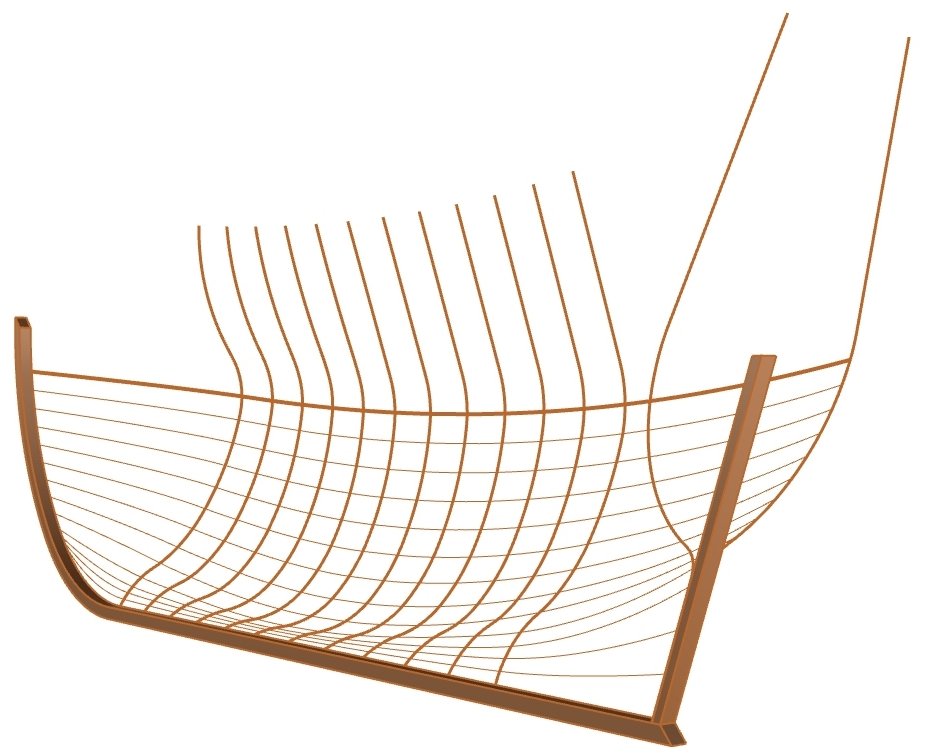
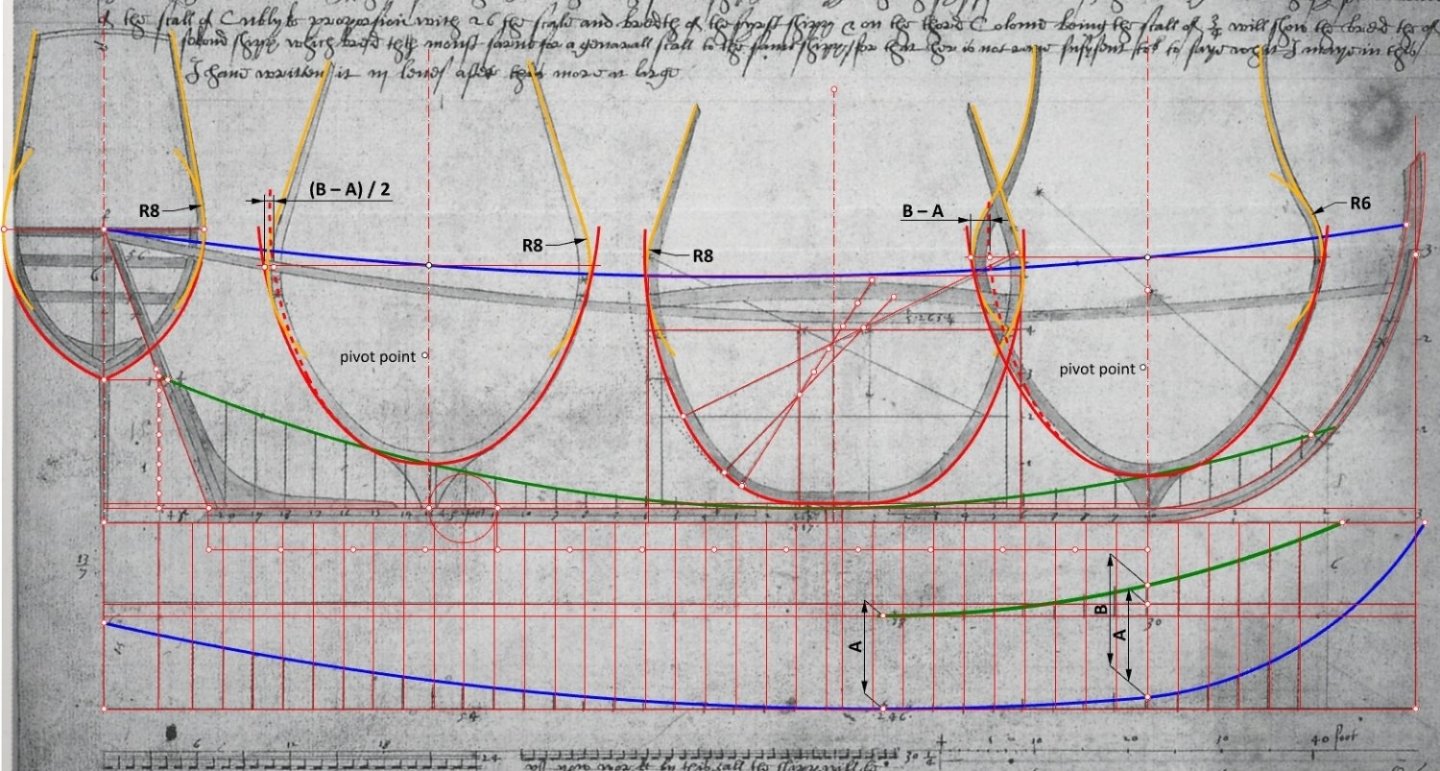
To make the method used more readable, slightly more detailed diagrams follow. For each section, the futtock template was moved vertically and horizontally (the position of the template after this operation is shown with a dashed line) and then rotated. The other sections (bends), closer to the ends of the ship, were already shaped by longitudinal ribbands. The hollowing and top timbers lines are not shown in the diagrams so as not to impair their legibility. Besides, from a conceptual point of view, these lines were of much less importance. -
EDIT: The conclusions expressed in this thread are out of date, the result, as it later turned out, of a misleading bit of material published in the official monographic publication on Mary Rose. The analysis and conclusions representing my current state of knowledge are contained in the thread: Apologies, and thank you, Waldemar Gurgul In the pursuit for answers, I have also decided to verify the body sections of the iconic ship Mary Rose, one of the first large vessel built in England using the skeleton method. So far, it has been assumed that the applied concept of the pre-designed frames formation in this ship was derived from some local tradition of unexplained origin, despite the fact that such a hypothesis contradicted the rationale from other historical findings and the overall picture of period shipbuilding. For more on this subject, see especially Mary Rose. Your Noblest Shippe. Anatomy of a Tudor Warship, 2009. However, a quick verification of the hull lines leaves not the slightest doubt that the ship was shaped according to the Mediterranean method, and in its most advanced form, i.e. using the futtock mould rotation/tilting (I posted a little more about this method in the thread "Mathew Baker's early concept of ship hull design, ca. 1570"). As can be seen from the graphics below, applying the Mediterranean method allows for an excellent rendering of the frames original shape in the midship part of the ship, taking into account, of course, the distortion of the original lines for various reasons.
-
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mathew Baker's early concept of ship hull design, ca. 1570
Waldemar replied to Waldemar's topic in Nautical/Naval History
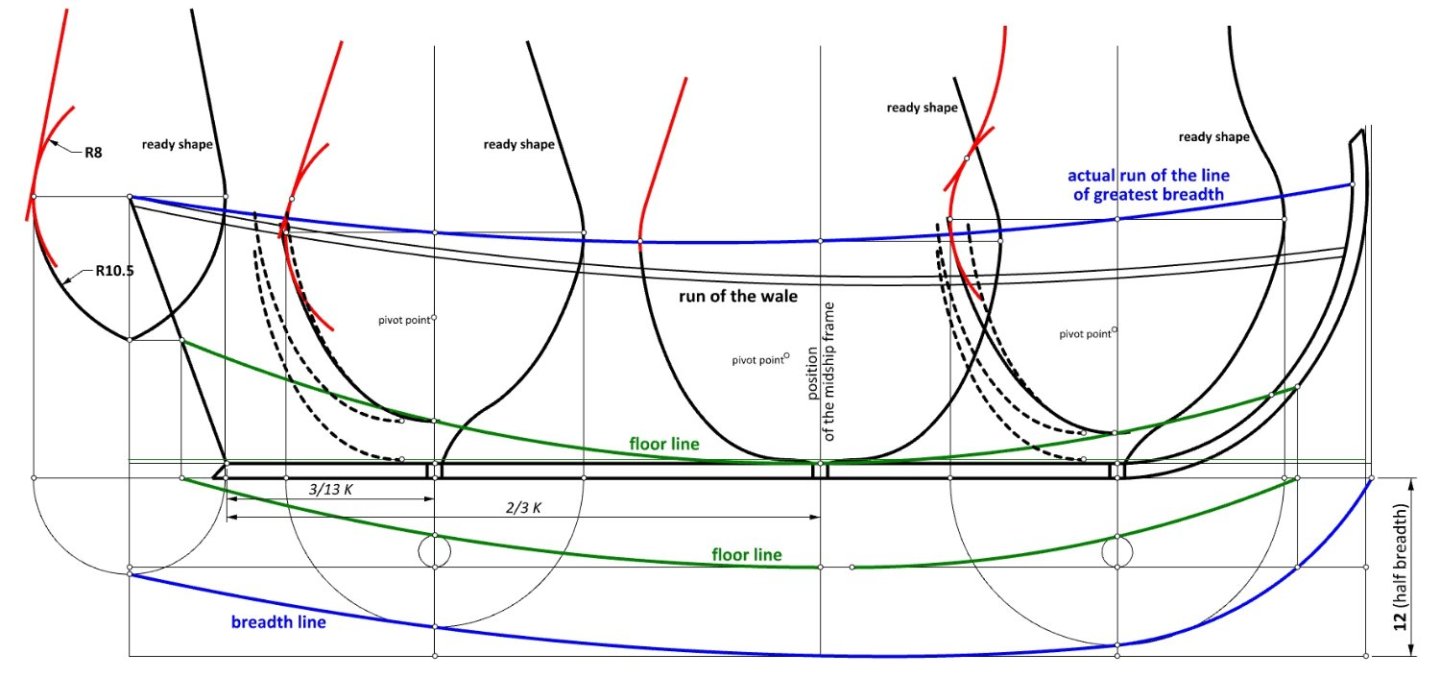
In the Danish archives there is another interesting 'complete' plan of a ship with a keel length of 68 feet, design breadth of 21 feet and the depth in hold of 9.5 feet. Its draft of 8 feet, written and drawn on the plan, is well suited for shallow Danish and Baltic waters. Contemporary descriptions on the drawing are in English, and, as in Baker's drawings taken from his Fragments, here too the ship is actually wider than the design grid. For this reason, I carried out a reconstruction of its hull shape using the Mediterranean method. Yet, interestingly, in this case the run of both narrowing lines was chosen in such a way that rotating the futtock moulds was not needed at all, only sliding vertically and horizontally is enough. Either way, the actual line of greatest breadth (of the reconstructed shape) does not coincide with the designed narrowing line of greatest breadth all along the length of the hull. I have shown both lines in different colours in one of the renders below. It is difficult to say who created this plan, but it is known that there were a few shipwrights of Scottish and English origin working in Denmark during this period. As can also be seen from both examples (i.e. from this one and Baker's), the Mediterranean method allowed for nice, smooth hull lines to be easily achieved, which certainly made it very popular. -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mathew Baker's early concept of ship hull design, ca. 1570
Waldemar replied to Waldemar's topic in Nautical/Naval History
Thank you for watching so far. Should anyone wish to mention in their publications or refer to this interpretation of Baker's drawing, my name is Waldemar Gurgul. -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mathew Baker's early concept of ship hull design, ca. 1570
Waldemar replied to Waldemar's topic in Nautical/Naval History
While rotating a wooden pattern, even of the most complicated shape, is extremely easy and convenient in full scale on the mould loft, the opposite is true when it comes to draw such a shape on paper by conventional methods in multiple copies, and each copy at a slightly different angle (using a paper template in scale would be not quite practical either). Therefore, when design plans on paper came into use in England, the Mediterranean method of rotating/tilting the futtock mould had to be substituted by a more suitable way. There is also one telling comment by Baker himself about his fellow shipbuilders, that they did not understand the purpose of rotating the moulds. Even if this remark may be a little unfair, it quite clearly suggests that English shipwrights quickly developed or used other methods. -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mathew Baker's early concept of ship hull design, ca. 1570
Waldemar replied to Waldemar's topic in Nautical/Naval History
To give an idea of how the pre-designed frames were shaped in full scale in this method, below an illustrative diagram. No (detailed) plans drawn on paper were needed, just few simple geometric scales/devices used on the mould loft. -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mathew Baker's early concept of ship hull design, ca. 1570
Waldemar replied to Waldemar's topic in Nautical/Naval History
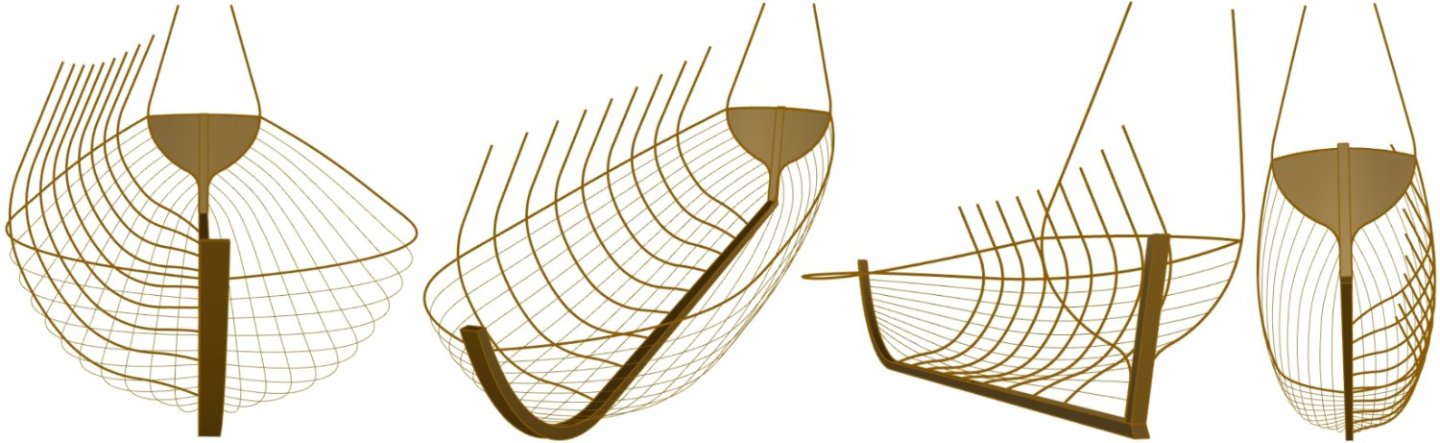
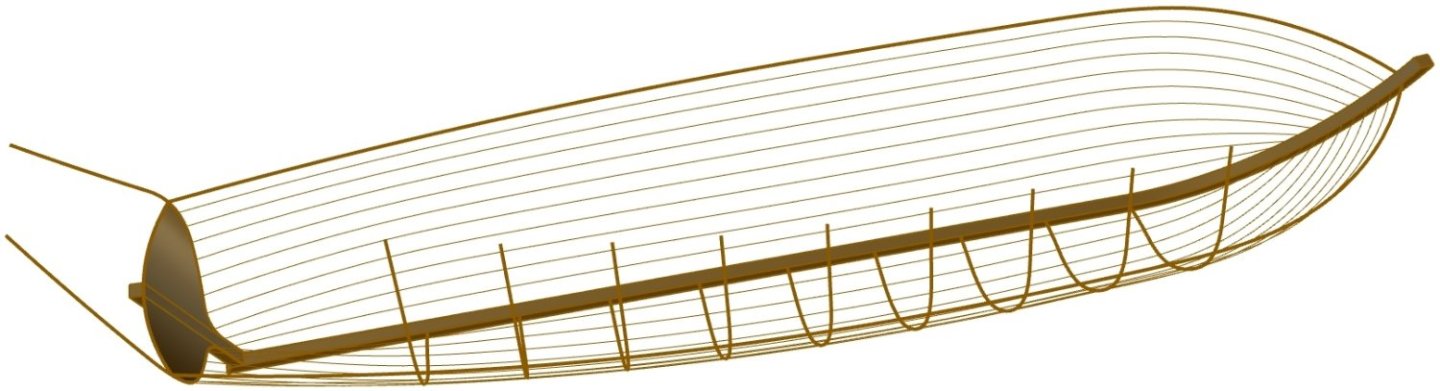
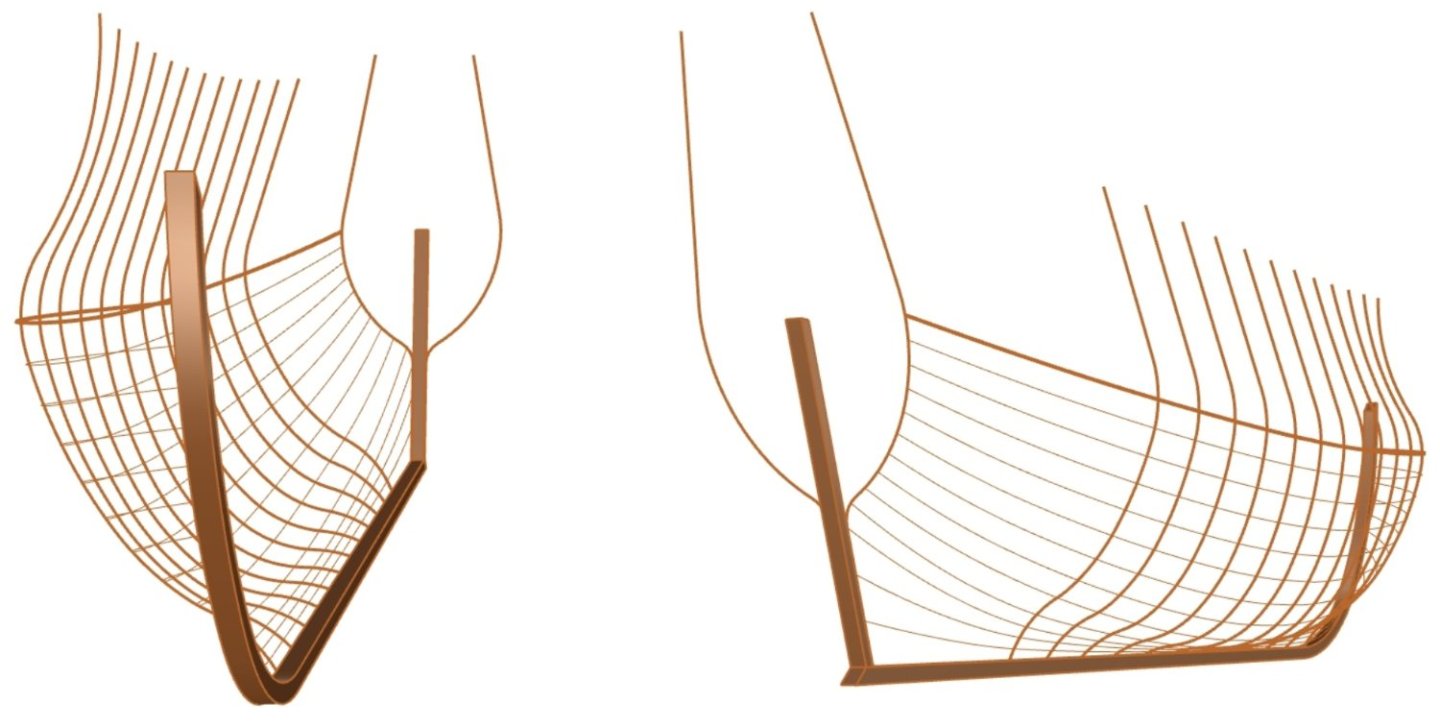
How exactly was this done? Many works have been written about the Mediterranean method, but by far the best single modern work is Le maître-gabarit, la tablette et le trébuchet. Essai sur la conception non-graphique des carènes du Moyen Âge au XXe siècle by Éric Rieth (1996). Below are some renders of a schematic 3D model of the ship drawn by Baker, made using the Mediterranean method. The pre-designed frames are located only between the quarter frames. The shape of the other frames, close to the ends of the ship, was determined by longitudinal ribbands. These elements can be seen in the graphics below. The overall shape and specificity of the lines obtained bear a striking resemblance to the hull shape of an Iberian whaling ship, the famous Red Bay vessel. -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mathew Baker's early concept of ship hull design, ca. 1570
Waldemar replied to Waldemar's topic in Nautical/Naval History
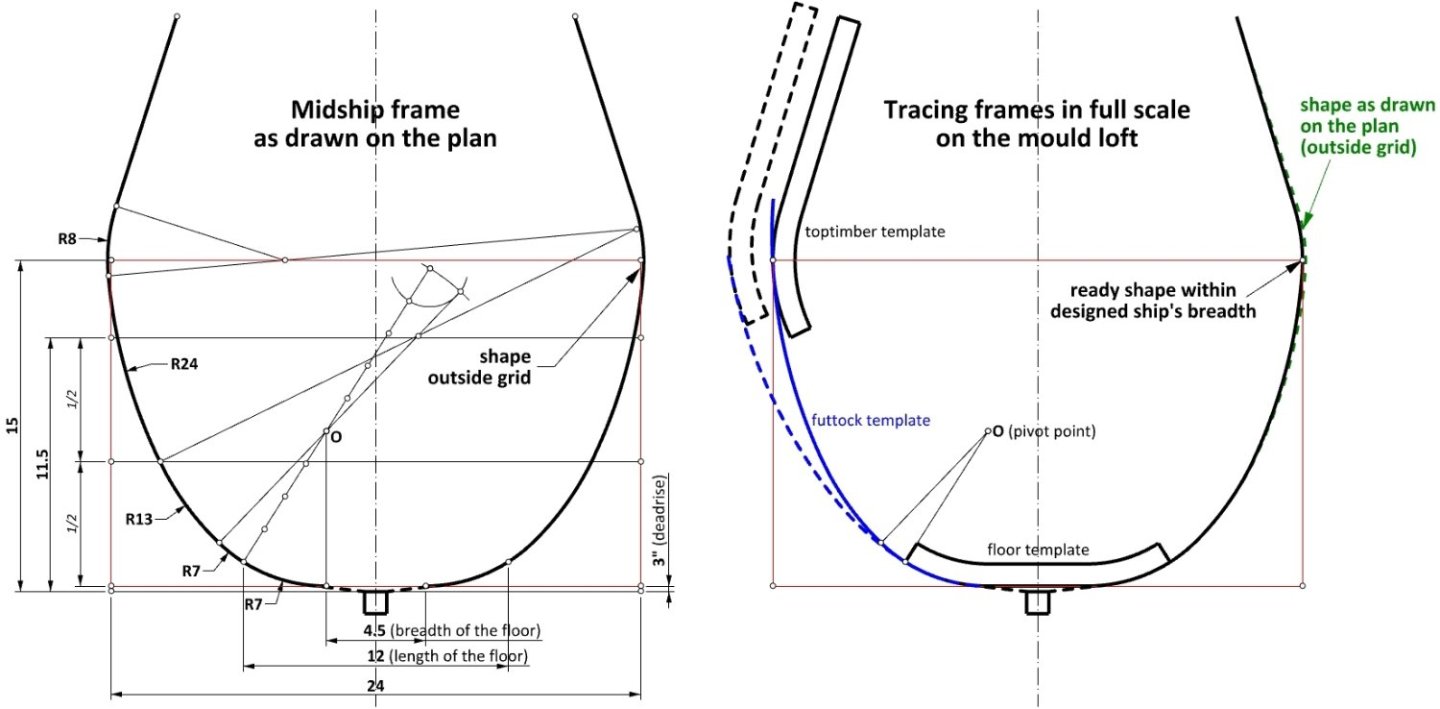
The following reproduction of a circa 1600 plan (from the Russian archives), possibly made by David Balfour, also confirms that contemporary designers did not treat the deadrise as an integral part of the designed frame shape, but rather as a necessary add-on. Again, the deadrise is outside the design grid, unlike all the rest of the frame profile. It is fair to say that a similar approach is taken by modern researchers, forgetting or deliberately omitting deadrise curves from their graphical reconstructions. Therefore, when conducting one's own research, one cannot rely only on modern interpretations of historical material. -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mathew Baker's early concept of ship hull design, ca. 1570
Waldemar replied to Waldemar's topic in Nautical/Naval History
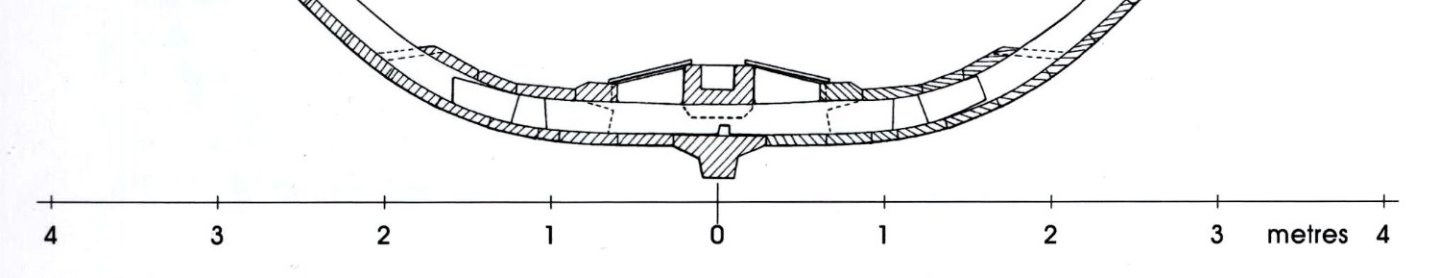
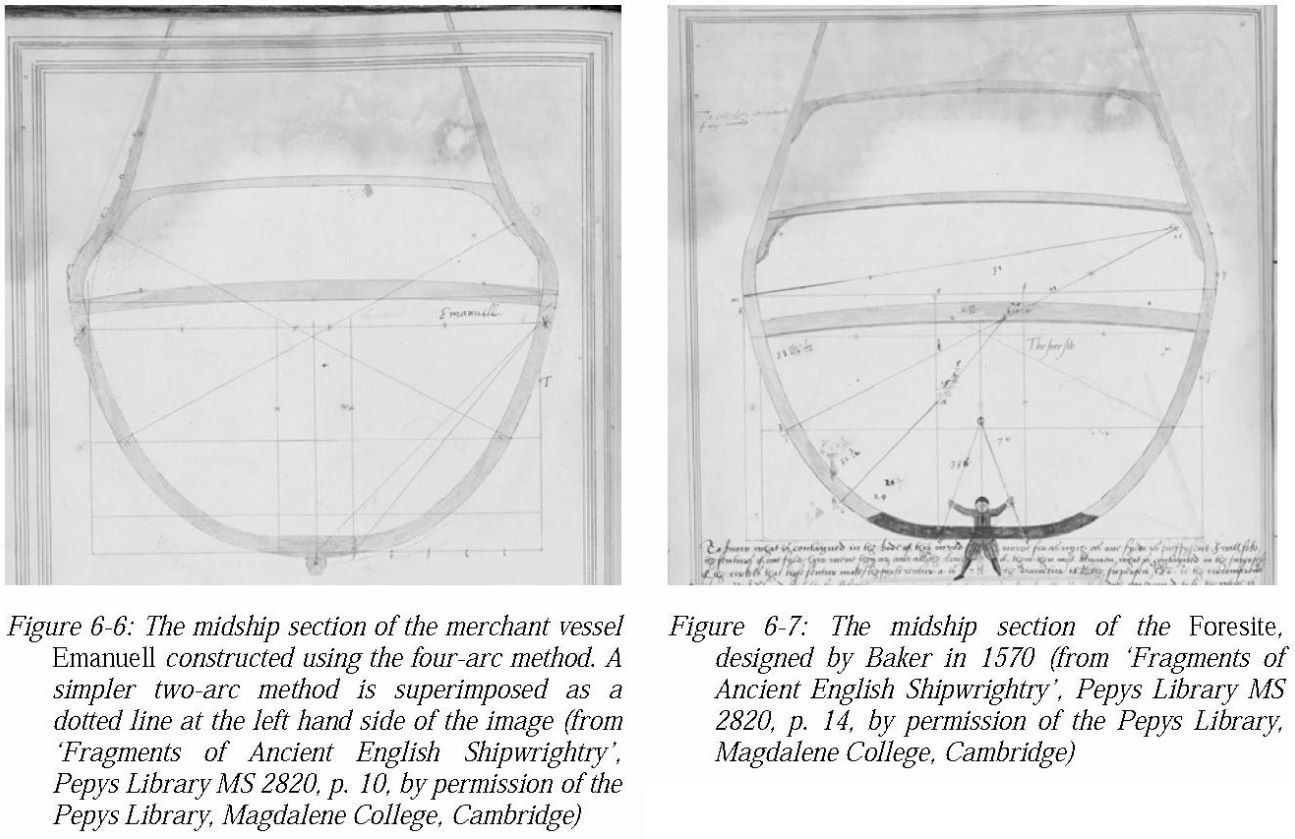
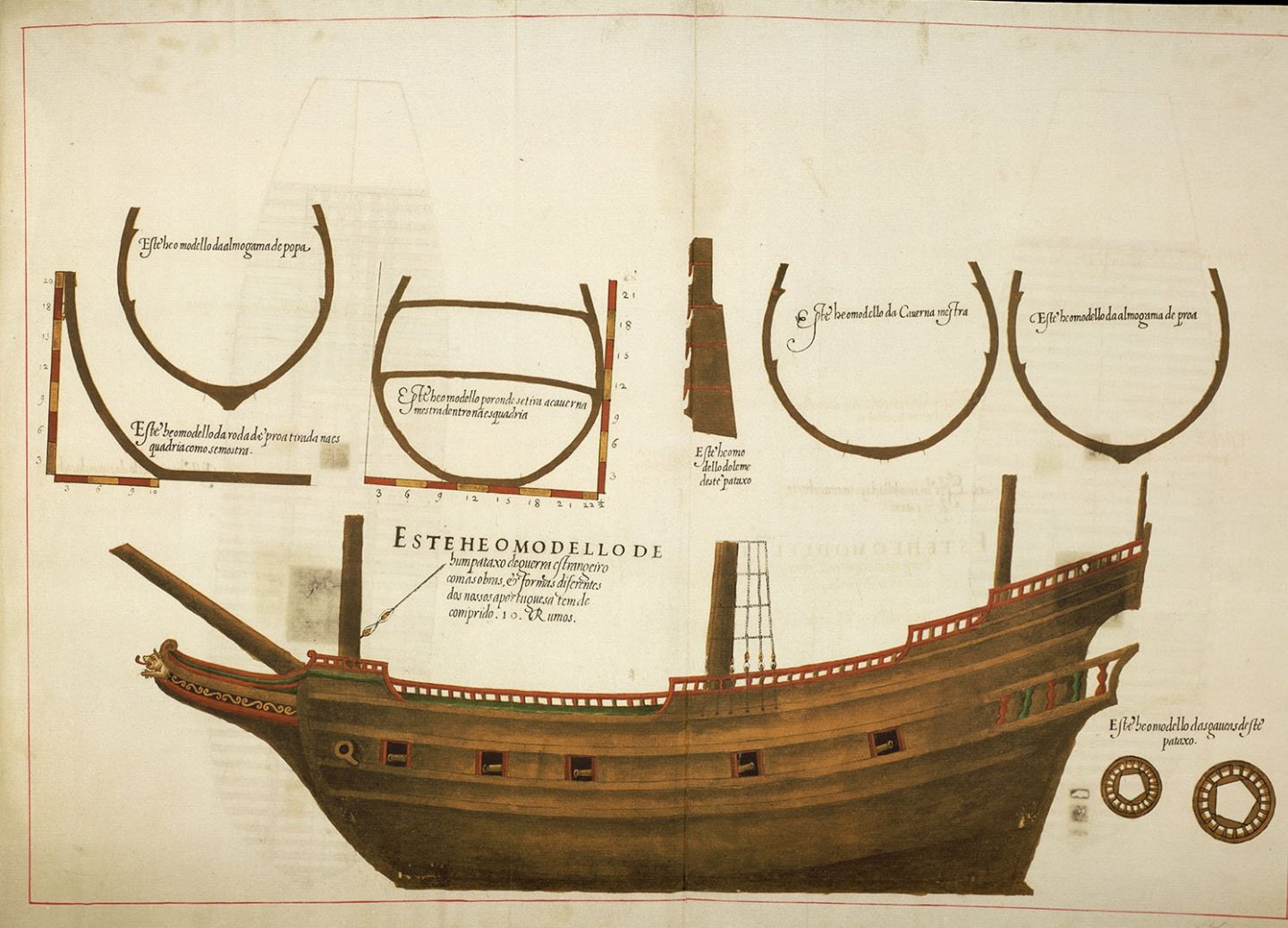
It will also not be out of place to show some examples found in shipwrecks and other written sources. It is clear that the deadrise curves for the main frame could be either concave or convex (the latter even mentioned in the Newton manuscript). Mary Rose (from The Tudor Warship Mary Rose by Douglas McElvogue): Basque whaling ship (from The Underwater Archaeology of Red Bay. Basque Shipbuilding and Whaling in the 16th Century, 2007). In this case the weakening of the floor timbers was to some extent compensated for by the T-shaped keel: Two examples of midship moulds from Baker's manuscript, the one on the left with the deadrise drawn in (from The Gresham Ship Project. A 16th-Century Merchantman Wrecked in the Princes Channel, Thames Estuary. Volume I: Excavation and Hull Studies, 2014): An instructive example also comes from the manuscript by the professional shipbuilder Manoel Fernandez, Livro da Traças de Carpintaria (1616). As can be seen, he designed the midship frame without the deadrise. It was then added later as a kind of supplement in a ready frame (compare two frames shown in the center of the draught). And some other archaeological examples or taken from shipbuilding period manuals, as drawn by me a few years ago: -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mathew Baker's early concept of ship hull design, ca. 1570
Waldemar replied to Waldemar's topic in Nautical/Naval History
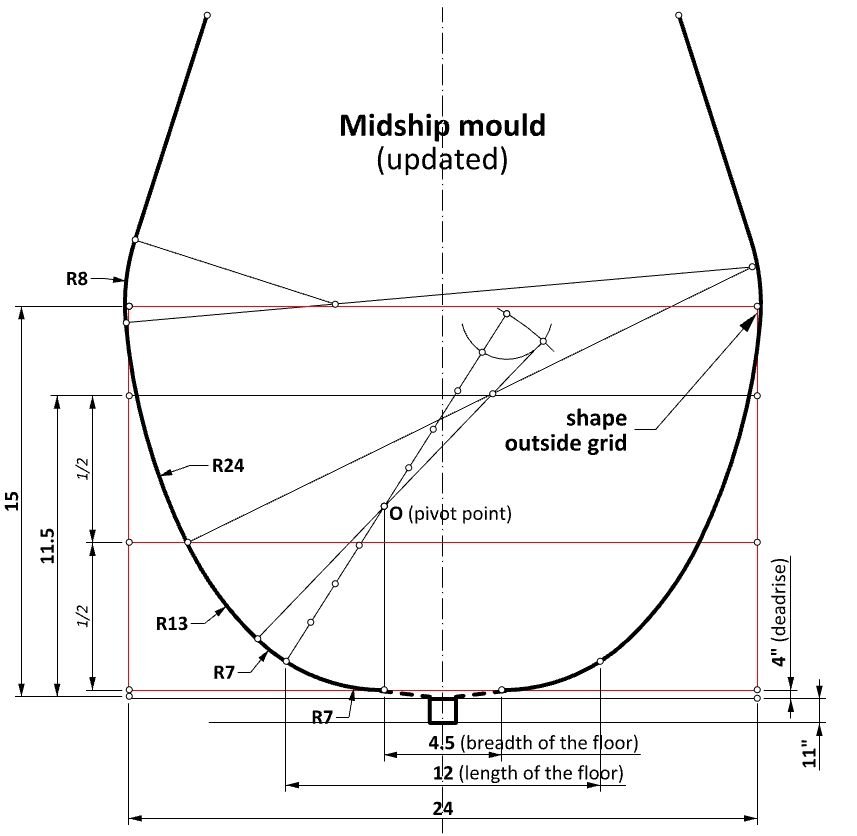
Hi Mark, Yes, this is all correct. Thanks to the improved quality of the reproduction of the Baker's plan, I was able to update the midship mould (shown below). As it happened, this only affects the deadrise, which was raised from 3 inches to 4 inches, at the expense of the keel height, reduced from 12 inches to 11 inches. Here I would also add that in the Harriot manuscript deadrise is specified for small ships at two inches and for large ships at three inches. I have also made other similar minor adjustments here and there, but these are without major impact on the overall reconstruction. For example, the keel length was increased from 60 to 60.5 feet. -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mathew Baker's early concept of ship hull design, ca. 1570
Waldemar replied to Waldemar's topic in Nautical/Naval History
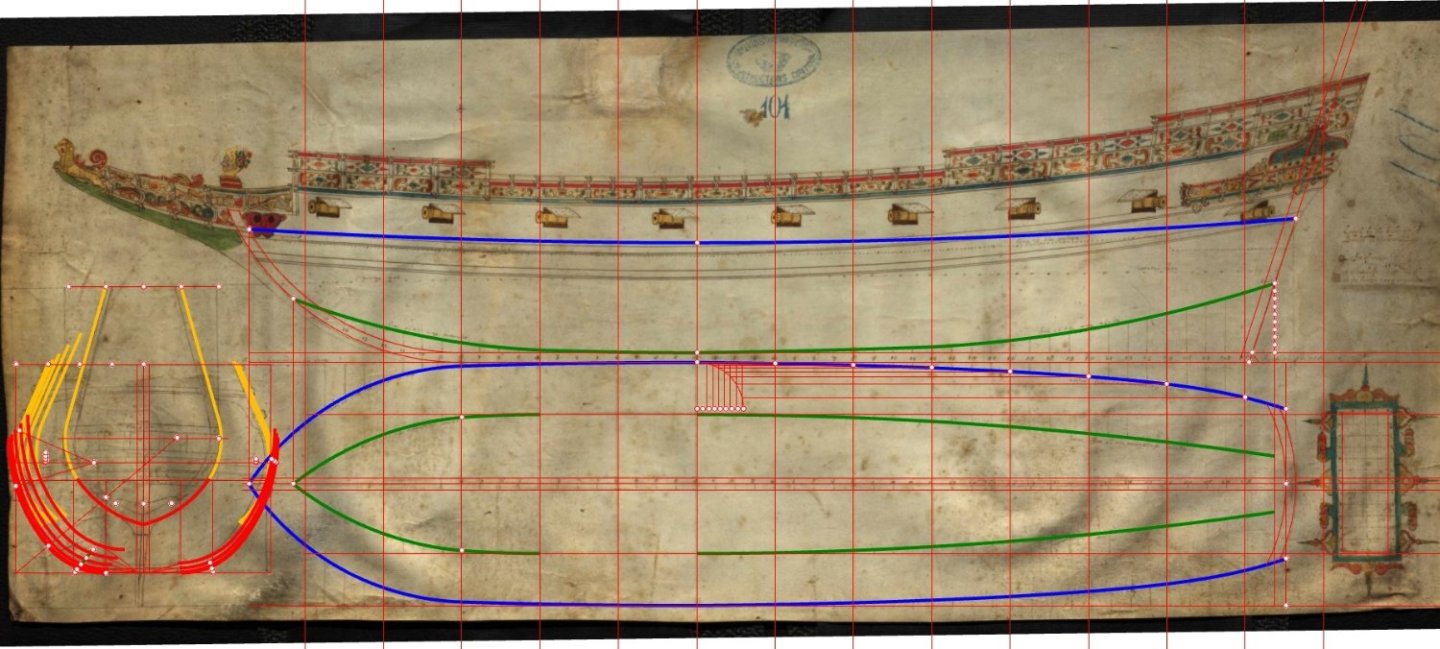
By a happy twist of fate, I found in my home resources a better copy of Baker's plan. This was decisive. After a few minor changes and amendments, the pure Mediterranean method clearly emerged. Taking into account inaccuracies of the original plan and to a lesser extent its later distortions, I find the resulting reconstruction of the lines satisfactory, finally revealing the method used by Baker. Two further detailed comments need to be added to the accompanying drawing. Baker deliberately did not draw the narrowing line of the floor for the stern section of the hull, because it was not necessary: he simply took the relevant coordinates for the stern quarter frame from the bow quarter frame. Of minor importance from the conceptual point of view, the radius of the breadth arc for the bow quarter frame is smaller than the rest. -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mathew Baker's early concept of ship hull design, ca. 1570
Waldemar replied to Waldemar's topic in Nautical/Naval History
A slightly different interpretation is also possible, closer even to the Mediterranean ways. The difference in the formation of the frame shape would be that the futtock template was moved and tilted first, and only then the toptimber mould (or the actual toptimber during skeleton assembly) was applied. This interpretation also explains well the slightly larger width of the actual ship compared to its design breadth. More clearly, this is the result of the Mediterranean method of frame forming combined with the adoption of a convenient round value for the futtock sweep radius. In addition, it may be related to the anomaly already mentioned that the position of the midship frame does not coincide with the greatest width of the hull. Normally, the Mediterranean method was used in a non-graphical way, i.e. without the use of a plan, so it is likely that Baker may have tried to sort it out in this way while making his drawing. It is now rather impossible to decide which of the two interpretations is correct (dimensional differences are rather negligible in this particular case). If one accepts the second, Baker's drawing would probably be the only drawing from the era that shows graphically the pure Mediterranean method. However, if one accepts the first interpretation, it could be considered to represent an intermediary between the Mediterranean method and the methods known from the slightly later other English manuscripts on shipbuilding. -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mathew Baker's early concept of ship hull design, ca. 1570
Waldemar replied to Waldemar's topic in Nautical/Naval History
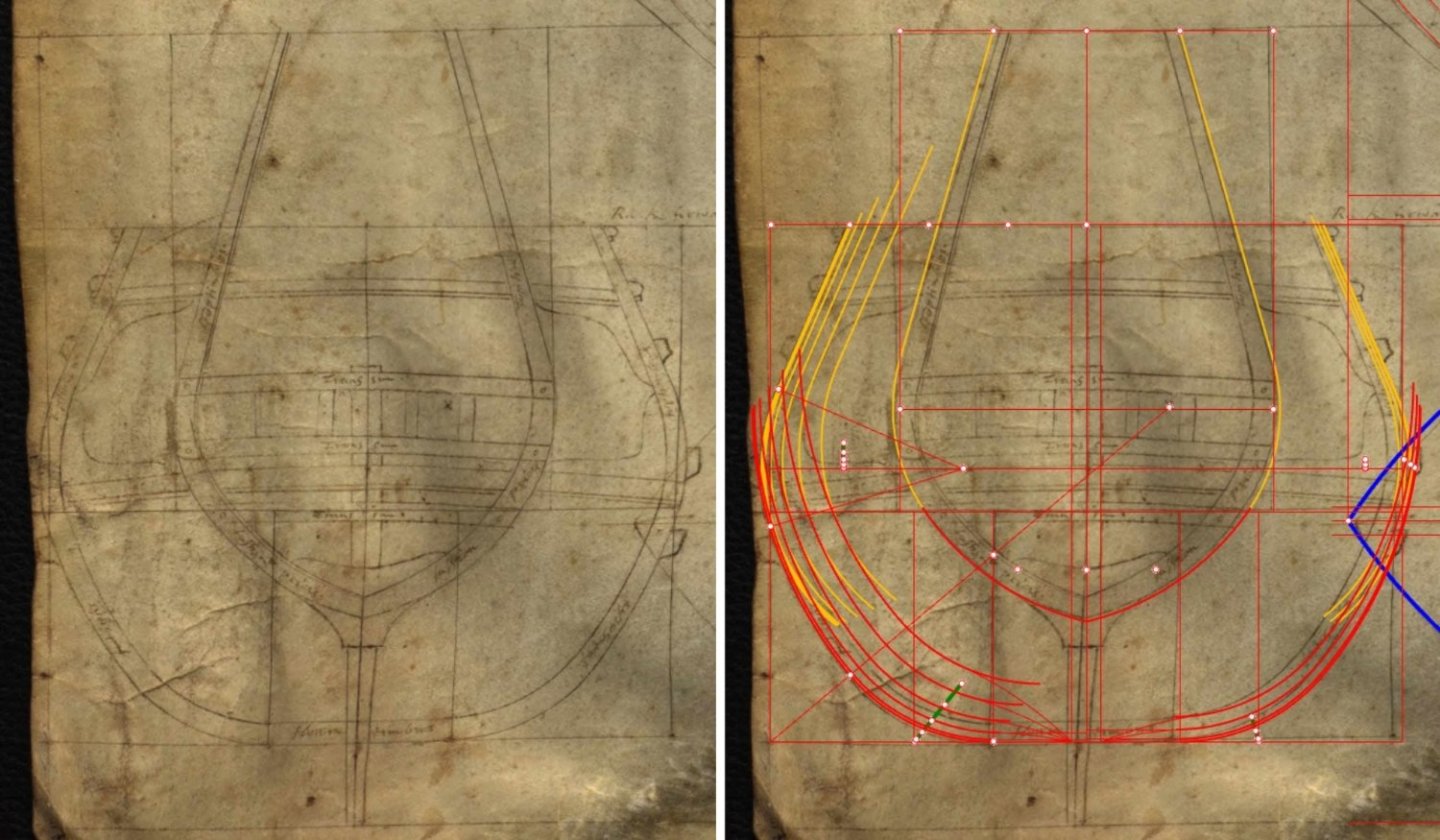
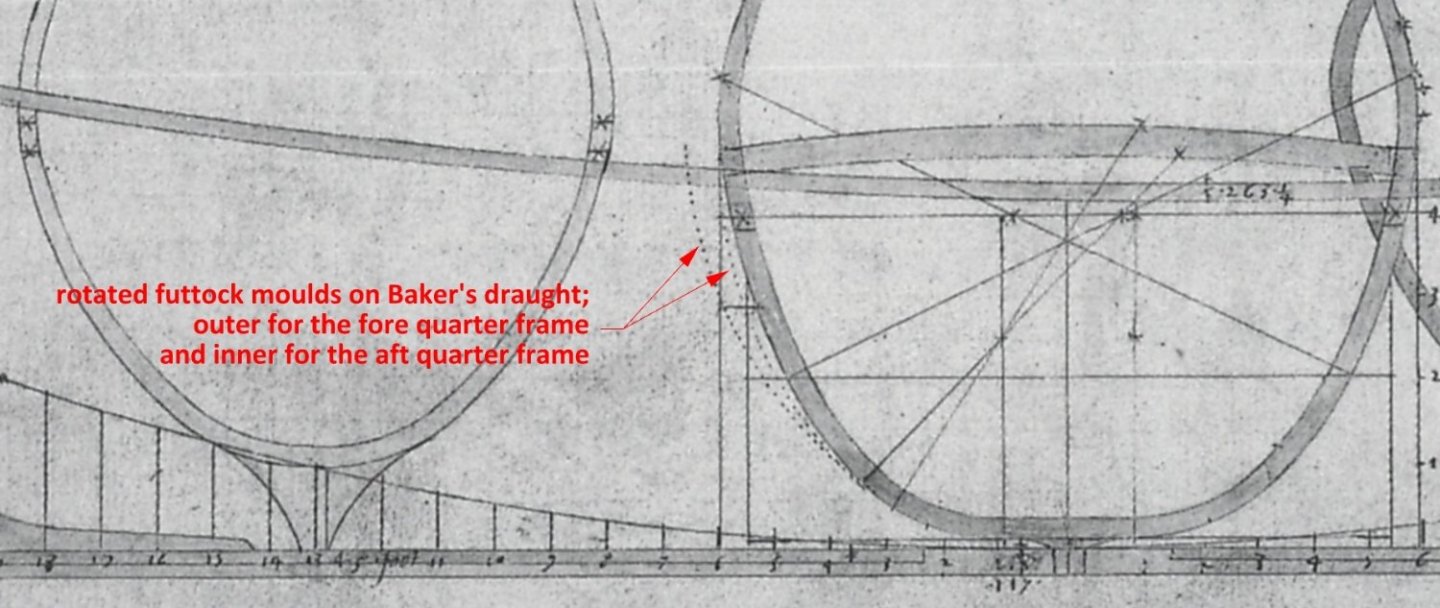
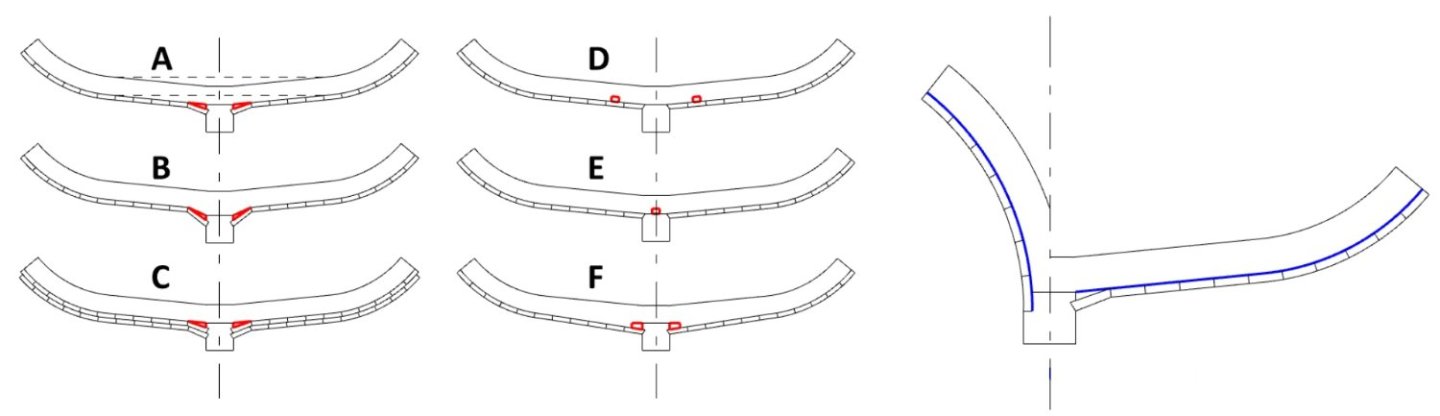
First, it should be said that the true line of greatest breadth was not drawn on the profile view or is invisible on the copy I am using. However, it could easily have been reconstructed thanks to the drawn profiles of the three frames in the original plan. This is the arc connecting the top of the sternpost to the quadrant point of the stempost, passing through the level of the greatest width of the frames. The accompanying drawing shows, in particular, the formation of the two quarter frames. The dashed lines show the successive positions of the futtock mould, moved vertically and horizontally according to the floor line coordinates, and then rotated to the point of contact with the arc of the greatest breadth. All the other frames in between the quarter frames could have been designed and constructed in exactly the same way, prior to their actual assembly into the hull under construction. The hollowing curves at the bottom of the frames have a rather careless character of quickly drawn single arcs to save time. -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mathew Baker's early concept of ship hull design, ca. 1570
Waldemar replied to Waldemar's topic in Nautical/Naval History
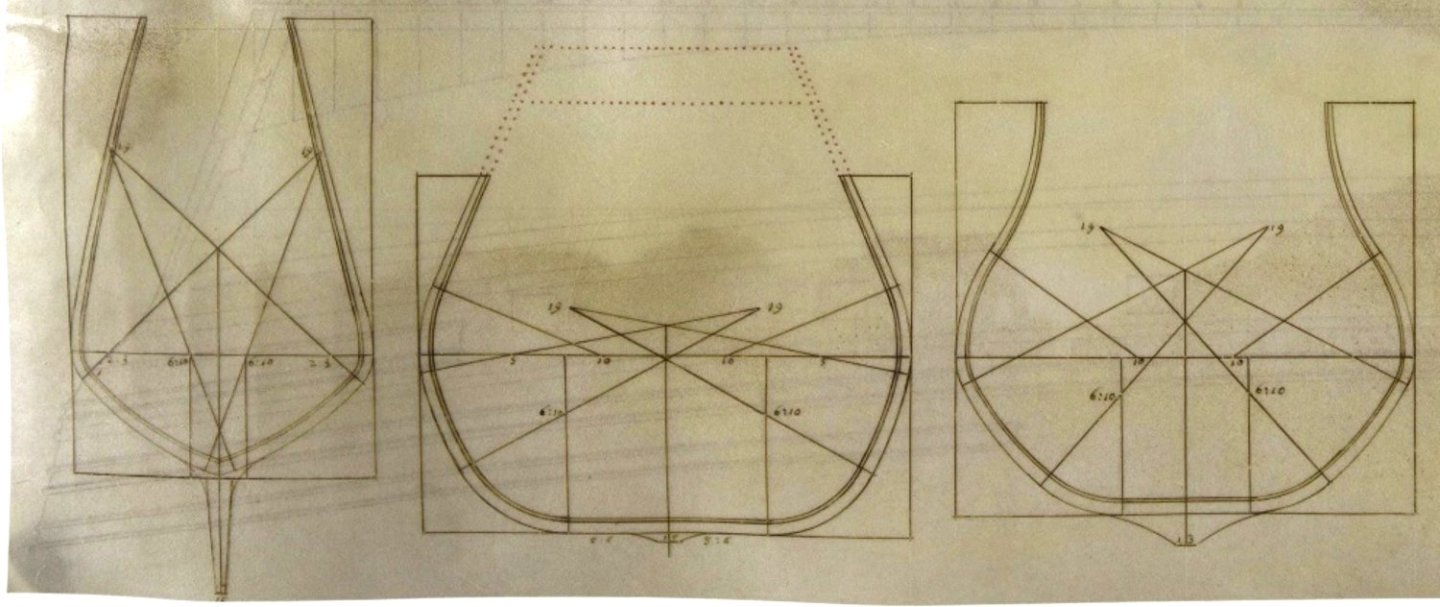
Okay. I guess all the design ambiguities have been recognised, despite the lack of appropriate commentary on this drawing in the manuscript. What follows is a reconstruction of the main frame, featuring two details that have not yet been recognised or convincingly explained in modern works on early English ship design. Firstly, the profile of the main frame in the drawing is slightly wider than the design breadth of the ship, but the quarter frames are already of normal width, in accordance with the plan view. The same phenomenon can be also observed in some other early ship draughts. This is due to the specifics of draughtsmanship and is a kind of a drawing convention. Tracing the frames in full-scale on the mould loft removes this inconsistency with the result of a 'normal' breadth. The second interesting detail is two adjacent arcs of the same radius. This is not a drawing mistake, as one of these arches is part of the floor timber (template) and the other is part of the futtock timber (template). This has to do with the conceptual method of shaping the profiles of the pre-designed frames very widespread in the Mediterranean. In this method, part of the frame profile was moved vertically and horizontally and then corrected by a slight rotation (fr. le trébuchement, span. joba). Baker must have learned this method during his Mediterranean travels and from Venetian immigrants. Ultimately, however, the method did not catch on in England, and no early English source work on shipbuilding describes this method. -
.thumb.jpg.c6343966b029e7941df5b987d129aac6.jpg)
Mathew Baker's early concept of ship hull design, ca. 1570
Waldemar replied to Waldemar's topic in Nautical/Naval History
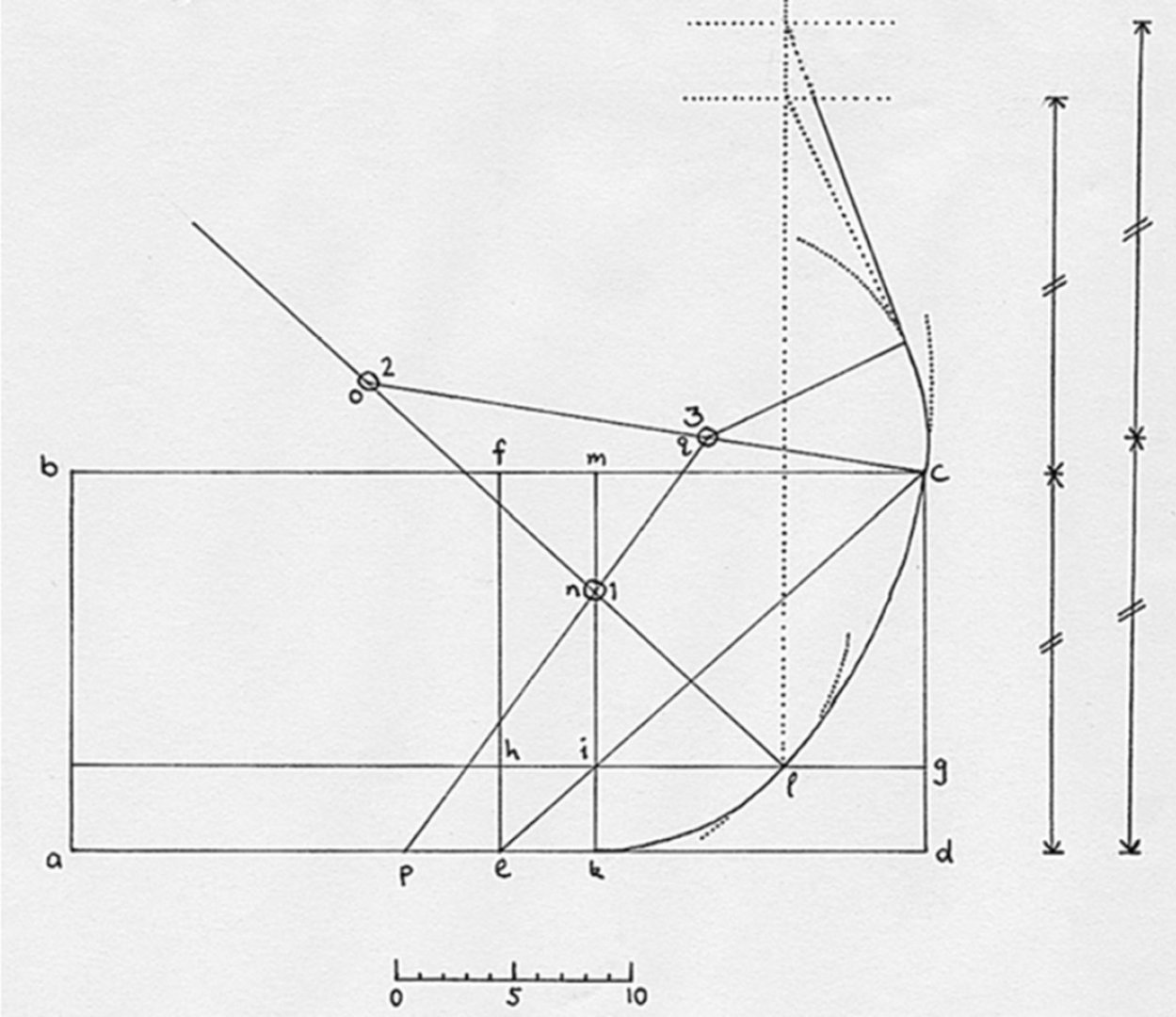
And there is no need to wait. The geometric construction of the midship mould on page 35 of the manuscript has already been explained by Johnston Stephen in Mathew Baker and the Art of the Shipwright, 1994. It is different from the midship mould of the ship plan I am analysing, so it cannot be used in this reconstruction: Figure 3.6. Fragments, p. 35 (redrawn). The diagram is a simplified version of Baker’s drawing. There are many more inked and scribed lines in the original, as well as numbers for the calculation of areas. In this example of Baker’s procedures for drawing the midship mould, breadth and depth are given as 36ft and 16ft respectively. dg = 1/5 ed. With eh = dg, draw gh. Then draw ec, cutting gh at i. Through i draw mk perpendicular to ed; ek is the floor for this half of the mould. Mark point l on gh such that hl = 2/3 gh. The first centre n is on mk and has its arc passing through k and l. Extend line ln beyond n; the second centre o is found on this extended line and its arc sweeps from l to c. To find the third centre, first mark the other half of the floor with p. The third centre q is at the intersection of oc and pn (extended). Baker then draws the upper futtock in three different ways.
About us
Modelshipworld - Advancing Ship Modeling through Research
SSL Secured
Your security is important for us so this Website is SSL-Secured
NRG Mailing Address
Nautical Research Guild
237 South Lincoln Street
Westmont IL, 60559-1917
Model Ship World ® and the MSW logo are Registered Trademarks, and belong to the Nautical Research Guild (United States Patent and Trademark Office: No. 6,929,264 & No. 6,929,274, registered Dec. 20, 2022)
Helpful Links
About the NRG
If you enjoy building ship models that are historically accurate as well as beautiful, then The Nautical Research Guild (NRG) is just right for you.
The Guild is a non-profit educational organization whose mission is to “Advance Ship Modeling Through Research”. We provide support to our members in their efforts to raise the quality of their model ships.
The Nautical Research Guild has published our world-renowned quarterly magazine, The Nautical Research Journal, since 1955. The pages of the Journal are full of articles by accomplished ship modelers who show you how they create those exquisite details on their models, and by maritime historians who show you the correct details to build. The Journal is available in both print and digital editions. Go to the NRG web site (www.thenrg.org) to download a complimentary digital copy of the Journal. The NRG also publishes plan sets, books and compilations of back issues of the Journal and the former Ships in Scale and Model Ship Builder magazines.